The most general form of a mirror in one dimension is a differentiable function $f:\mathbb R \rightarrow \mathbb R$, for which $f(x)$ determines the vertical position of the mirror at displacement $x$ and $f'(x)$ determines the slope and hence the angle of the mirror. The simplest examples of such mirrors are plane mirrors defined by $f(x)=c$ where $c$ is a constant, and parabolic mirrors defined by $f(x) = x^2/4a$ where $a$ is the focal length of the mirror.
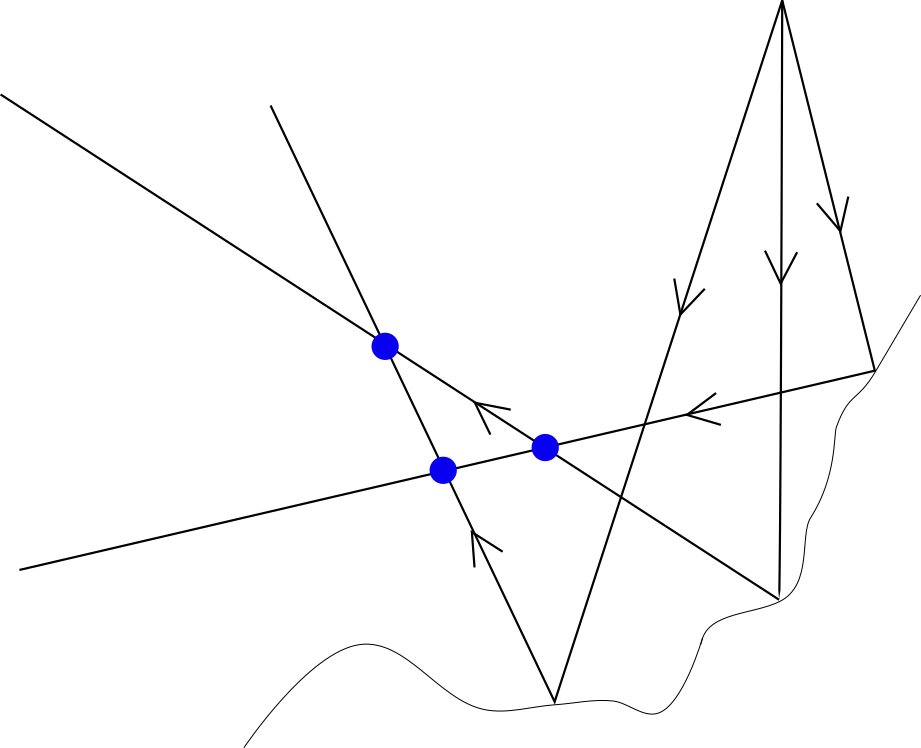
Furthermore, for an object at some position in front of the mirror, an image is only formed if all rays that reflect once off the mirror intersect at one point (here I am referring to the continuations of the rays into lines, as is done when finding the position of a virtual image). It is relatively easy to prove that, for any object position, a plane mirror forms a valid image. However, I have not yet been able to prove so for a parabolic mirror, and for a general mirror the task seems formidable. Also, a general mirror would not necessarily form an image for any object; consider the image below, for example, where three rays from one point reflect and intersect at three distinct points. Hence, we should not be able to prove that every mirror always forms an image.
As such, I was wondering if there is a general set of criteria that determines whether or not some mirror will form an image for any object distance. It seems like a pretty important problem, and I haven't been able to find any discussion of it anywhere (then again, I'm not great at googling so I might have just missed it).