Full disclosure, this answer is based on a paper I recently published on this topic. The paper's title, "Defining the temperature of an isolated molecule," gives away that my answer is yes, we can define such a temperature.
Indeed, the concept of the temperature of an isolated molecule in a vacuum isn't new. It's been around for decades in fields such as astrophysics and molecular beam collisions (I give many references in the paper).
For instance, PAH (polycyclic aromatic hydrocarbons) were discovered in interstellar media in 1984 when K. Sellgren assigned certain near-IR features appearing in the spectrum of several nebulae to the "thermal emission from very small grains (radius 10 Å) which are briefly heated to ~1000 K by absorption of individual ultraviolet photons." Such grains turned out to be large PAH molecules.
To define the temperature, we must consider that an isolated molecule is a microcanonical system conserving total energy. (At least in the short term, as it slowly irradiates the energy excess as a black body.) Therefore, we work with microcanonical temperatures rather than conventional canonical temperatures. The main distinction is that the microcanonical temperature is a function $T\left(E\right)$ of the total energy $E$, while the canonical temperature is the opposite; the energy $E\left(T\right)$ is a function of the temperature.
The problem of defining the microcanonical temperature of an isolated molecule boils down to whether it's possible to compute $$ T={{\left( \frac{\partial S}{\partial E} \right)}^{-1}} $$ for a finite system with a few degrees of freedom. In this equation, $S$ is the entropy, which, according to Boltzmann, is $$ {{S}_{B}}\left( E \right)={{k}_{B}}\ln \left[ \varepsilon \,\omega \left( E \right) \right],$$ where $ \omega\left( E \right) $ is the density of microstates with energy $E$.
In principle, the discrete nature of $E$ for a small, finite quantum system can pose significant troubles for computing the derivative defining the temperature. However, numerical tests show that as long the energy excess is not too small (near the zero-point level), the $ \omega\left( E \right) $ is nicely differentiable.
Then, we can go on. We can compute $ \omega\left( E \right) $ for an isolated molecule numerically, get the derivative, and have its microcanonical temperature. If we are happy with a harmonic approximation for the normal vibrational modes, we can even get $ \omega\left( E \right) $ analytically and write a nice closed expression to $T\left(E\right)$.
We have just to consider another problem before: the entropy definition is not unique. According to Gibbs, for instance, the entropy should consider all microstates with energy small or equal to $E$, thus, it should be $$ {{S}_{G}}\left( E \right)={{k}_{B}}\ln \left[ \Omega \left( E \right) \right], $$ where $\Omega \left( E \right)$ is the integrated number of states.
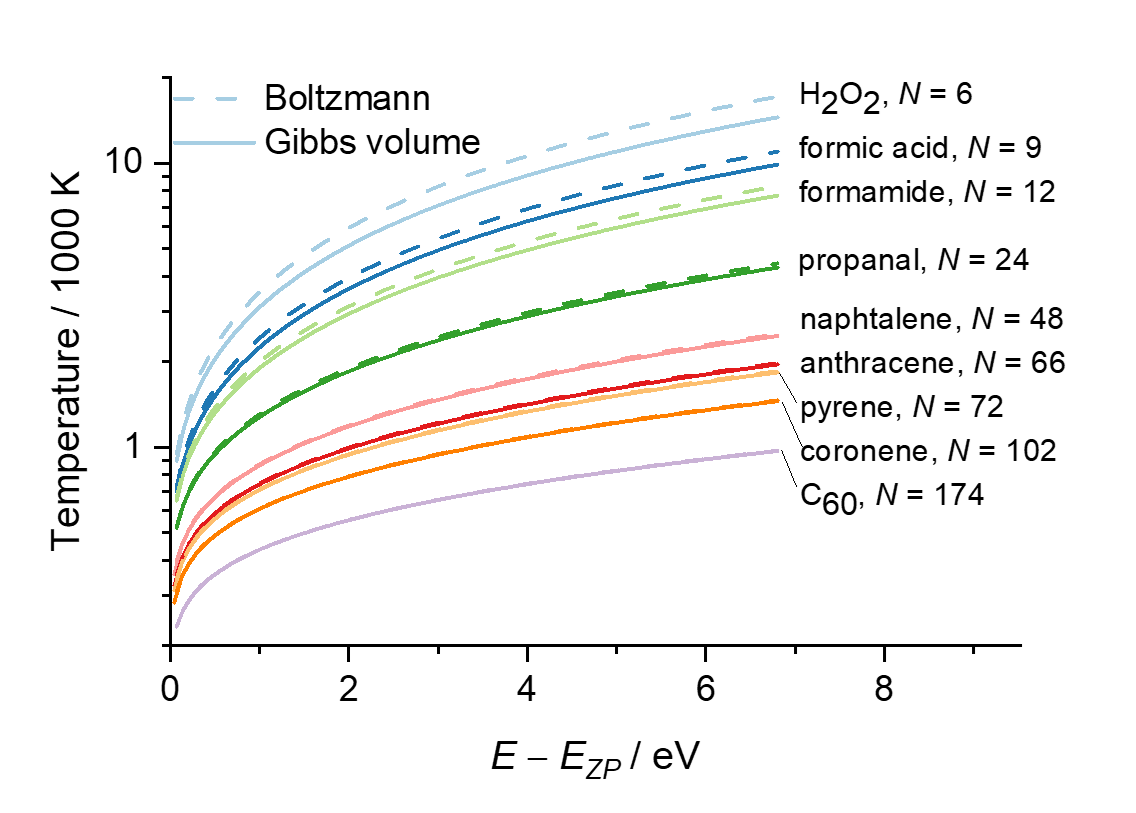
Both entropy functionals in the thermodynamic limit give the same results for large systems. However, for a small, finite system such as an isolated molecule, the predictions from both approaches may not match. Indeed, this is the case for molecules up to about ten atoms.
Anyway, for a large molecule, Boltzmann and Gibbs volume microcanonical temperatures agree well. You can estimate itthem with this simple equation $$ T\left(E\right)={{\left[ \ln \left( \frac{E+{{E}_{ZP}}}{E-{{E}_{ZP}}} \right) \right]}^{-1}}\frac{2{{E}_{ZP}}}{N{{k}_{B}}}, $$ where $E_{ZP}$ is the harmonic zero-point energy and $N$ is the number of vibrational degrees of freedom $\left(N = 3N_{atoms}-6\right)$.
The figure below shows a few examples of the microcanonical temperature of isolated molecules in the harmonic approximation.