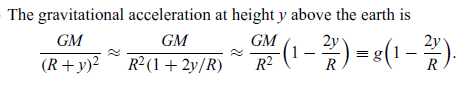The problem is to find the time it takes a particle dropped from a height $h_0$ above the surface of the earth to reach the surface (exactly, not approximately i.e. $g$-value is not constant, in the limit $h_0<<R$ where $R$ is the radius of the earth) assuming the earth to be a perfect sphere.
How I did it is as follows : Let the acceleration due to gravity at the surface of the earth be $g$. At a height $h$ above the surface of the earth, the acceleration due to gravity is $-\frac{gR^2}{(R+h)^2}$(considering upward to be positive), hence $$\ddot{h}=-\frac{gR^2}{(R+h)^2}\implies \dot{h}d\dot{h}=-\frac{gR^2dh}{(R+h)^2}\implies \frac{\dot{h}^2}{2}=gR^2\left(\frac{1}{R+h} -\frac{1}{R+h_0}\right)$$ Now $$\frac{dh}{dt}= \dot{h}=-\sqrt{2gR^2}\sqrt{\frac{1}{R+h} -\frac{1}{R+h_0}}\implies \sqrt{2gR^2}t=\int_{0}^{h_0} \frac{dh}{\sqrt{\frac{1}{R+h} -\frac{1}{R+h_0}}}=\sqrt{R+h_0}\left((R+h_0)\arctan\sqrt{\frac{h_0}{R}}+\sqrt{Rh_0} \right)$$ when $h_0<<R$, it may be written as $$\begin{align}\sqrt{2gR^2}t \approx \sqrt{R+h_0}\left((R+h_0)\sqrt{\frac{h_0}{R}}+\sqrt{Rh_0} \right)\implies t&=\sqrt{\frac{2h_0}{g}}\left(1+\frac{h_0}{2R}\right)\sqrt{1+\frac{h_0}{R}}\\&\approx \sqrt{\frac{2h_0}{g}}\left( 1+\frac{h_0}{2R}\right)^2 \\& \approx \sqrt{\frac{2h_0}{g}}\left(1+\frac{h_0}{R}\right) \end{align}$$
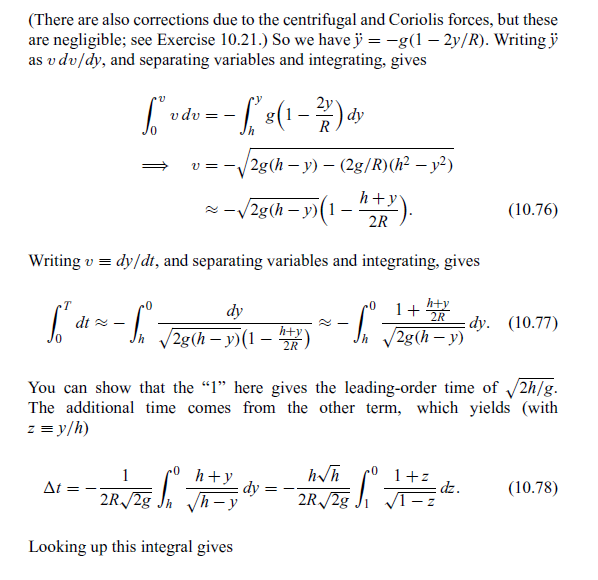
But it is not the answer, instead the answer is $t=\sqrt{\frac{2h_0}{g}}\left(1+\frac{5h_0}{6R}\right)$. The author gives the below solution and gets to this answer :
I want to know have I done something wrong? Or is this error due to chronology of taking approximations? Which answer is 'more' correct? I am very confused, any help is appreciated. Thanks