I'd really appreciate if someone could give a more descriptive answer on why Newtons Third Law does not cancel out.

The effect of the forces each object exerts upon the other depends on the application of Newton's second law, not the third law. Newton's second law says the net external force acting on a object equals its mass times its acceleration, or $F_{net}=ma$.

Or at least give a real life example of why it's the case.

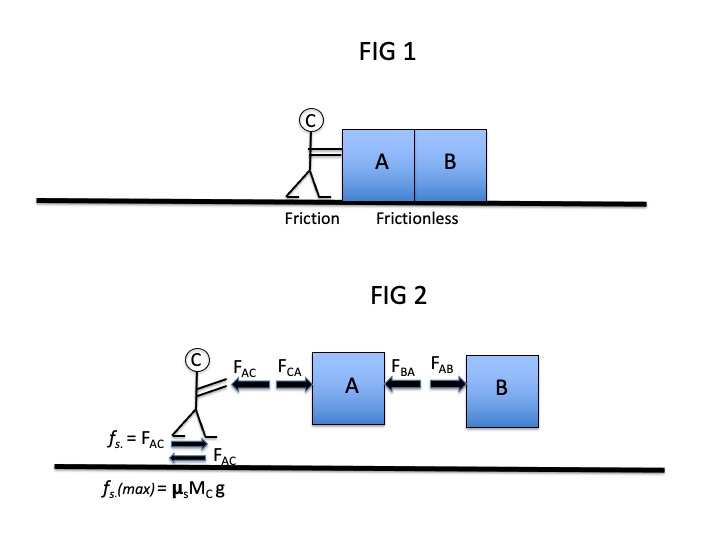
See FIG 1 below. A man stands on a surface having friction. Two blocks are in contact with each other are on a frictionless surface, or at least a surface with negligible friction (e.g. ice) compared to the surface the man stands on (e.g., dry pavement). The man applies a force to block A.

In order to analyze all the forces including all pairs of forces per Newton's 3rd law we draw a free body diagram of the man and each block per FIG 2 below. Note that the equal and opposite Newton 3rd law pairs of forces are (1) between the man and the ground, (2) between the man and block A, and (3) between block A and block B.

To determine the effect of these forces on the man, block A, and block B we need to apply Newton's second law to each individually.

Block B:

Note that the only external force acting on block B is the force exerted on it by block A, or force $F_{AB}$. From Newton's second law

$$F_{AB}=M_{B}a\tag{1}$$

Where $M_B$ is the mass of block B and $a$ is its acceleration.

Block A:

There are two external forces acting on block A. The force exerted on it by block B, $F_{BA}$ and the force exerted on it by the man, $F_{CA}$. Thus the net external force acting on A is $F_{CA}-F_{BA}$. From Newton's second law, realizing that since blocks A and B move together they will have the same acceleration $a$, we have

$$F_{NET}=F_{CA}-F_{BA}=M_{A}a\tag{2}$$

Adding equations (1) and (2) we obtain

$$F_{CA}=(M_{A}+M_{B})a\tag{3}$$

Which is the same as saying the only external force acting on the combination of blocks A and B is the force $F_{CA}$ exerted by the man giving the combination of blocks an acceleration of $a$ per Newton's 2nd law.

This example clearly shows that the equal and opposite forces A and B exert on one another, per Newton's 3rd law, do not "cancel" each other, because there is a net external force acting on the combination of A and B per Newton's 2nd law, causing both blocks to accelerate

So what about the man?

Man:

There are two external forces acting on the man, the force exerted by block A, $F_{AC}$ and the static friction force $f_{s}$ exerted by the ground on the mans feet. The static friction force $f_s$ that the ground exerts on the man is equal and opposite to the force the man exerts on the ground, another Newton's 3rd law pair.

The static friction force $f_s$ will match the force of block A $F_{AC}$ until the maximum possible static friction force is exceeded, in which case the man feet will slip. That would happen if the man pushed too hard on block A. We will assume he doesn't slip, in which case the net force on the man is zero and his acceleration is zero.

But the reason the man doesn't accelerate is not because the equal and opposite pair of forces between him and the ground or between him and block "cancel", but because the two external forces are equal and opposite for a net external force on the man of zero.

Hope this helps.