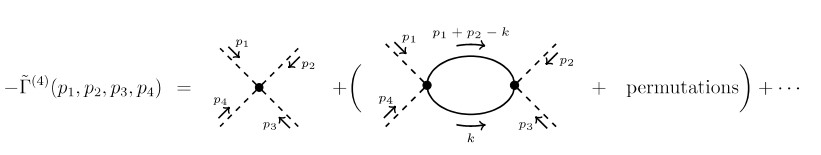
I am going through the lecture notes for my class and I can't seem to follow the logic. Maybe this is considered a homework problem, but I could not find anything that directly answers my question on the internet and thought the physics stack exchange is the best place to ask. As we know, $ \Gamma^{(4)}(p_1,p_2,p_3,p_4)$ has a superficial degree of divergence of zero. The lecture notes try to explain why $D = 0$ means it is logarithmically divergent. The notes consider the leading order Feynman diagrams:
Question 1: Looking at the diagram with 2 internal vertices, it seems to me that the momentum at each vertex should be conserved. Meaning if we let $k$ be the momentum flowing from the first vertex to the second as in the diagram, momentum conservation should give us two possible expressions for the momentum flowing through the top internal propagator.
Question 2: In addition, I assume the "+ permutations" means he is considering all four point irreducible diagrams with 2 internal vertices but could it possibly mean permutation of momenta? I don't know if permutation of momenta even makes sense since the diagram is still one integral.
If you consider vertex 1, $p_1 +p_4 -k$, or if you consider vertex 2, $-p_2-p_3-k$. However, I don't see how it could be $p_1+p_2-k$.
Now, the integral for the diagram is $$ -\lambda \int \frac{d^4k}{(k^2+m^2)((p_1+p_2-k)^2 + m^2)} $$
which for $|k| >> m$ and $|k| >> |p_1+p_2|$, it is approximately, $\int \frac{d|k|}{|k|}$. If a cut-off factor $\phi(k^2/\Lambda^2)$ is introduced, the integral should be approximately $\int \frac{\phi^2(k^2/\Lambda^2)d|k|}{|k|} = \int^\Lambda \frac{d|k|}{|k|} = log(\Lambda)$. However, the lecture notes claim the integral with cut-off factor will give $\frac{\lambda^2}{32 \pi^2}(log(\Lambda^2/m^2)+f(p_{12}))$. Where the lecture notes let $p_{12} = p_1 +p_2$.
Question 3: I don't understand why the integral would be $log(\Lambda^2/m^2)$ instead of $log(\Lambda)$ and how we know that the term $f(p_{12})$ that depends on external momenta can be written as a sum to the logarithmic term and not something like $log(\Lambda^2/m^2)f(p_{12})$. I do not know how to explicitly calculate the first integral to check using explicit calculation.