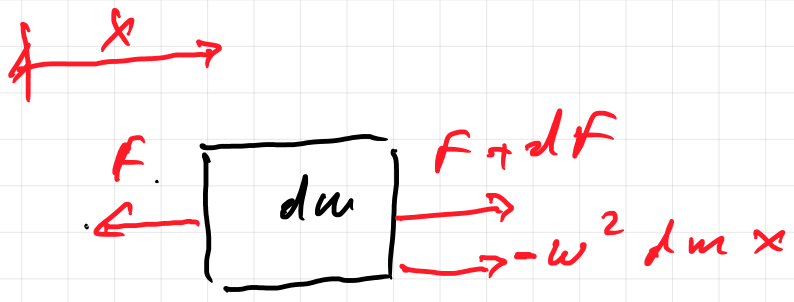
The "centripetal force" on a small mass element is
$$dF=dm\,\omega^2 \,x\\ \text{with}\quad dm=A(x)\,\rho\,dx=A\,\rho\,dx $$$$F+dF-F-dm\,\omega^2\,x=0\quad\Rightarrow\\dF=dm\,\omega^2 \,x\\ \text{with}\quad dm=A(x)\,\rho\,dx=A\,\rho\,dx $$
you obtain
$$F=A\,\rho\,\omega^2\int x\,dx=\frac 12 A\,\rho\,\omega^2\,x^2+c\\ \text{with}\quad F(L/2)=0\quad\Rightarrow c=-\frac 18\,A\rho\,{\omega}^{2}L^2$$
thus $$F(x)=\frac 12\,A\,\rho\,\omega^2\left(x^2-\frac{L^2}{4}\right) \quad, 0\le x\le \frac L2$$
you can obtain the angular velocity $~\omega~$ from the EOM
$$I_{\text{CM}}\,\dot\omega=\tau\quad,I_{\text{CM}}=\frac{m\,L^2}{12}$$