If the type of modulation you are talking about is FM, then the spectral content depends on its modulation index (M or $\beta$). The full equation for the output is (for a sinusoidal input only):
The term inside the cosine is not easy to isolate.
With some algebra it can be separated out using bessel functions:
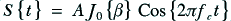
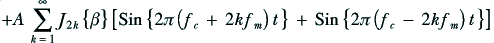
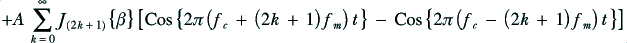
So you can see that this signal has frequency components at $f_c + n*f_m$. Where n is $-\infty$ to $+\infty$. Shocking isn't it? The Bessel Function $J_n\{\beta\}$ tends to approach zero at large values of n, but it is counter-intuitive to see all this frequency content.
So a simple sine wave modulation at frequency Fm can produce a variety of spectra depending on its modulation index (M or $\beta$) which is a ratio of the change in frequency deviation ($\Delta$f) to the maximum frequency of the modulated signal ($f_{m(max)}$). See the plots for different values of M for the same modulating tone at frequency $f_m$ where $M=\frac{\Delta f}{f_m}$
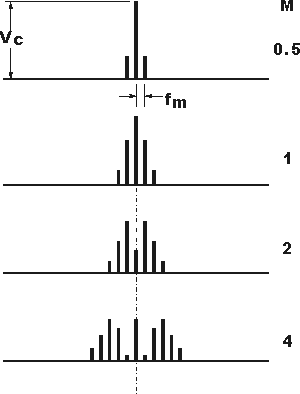
In reality, Carson's Rule is applied to limit the spectral content to about 98% of all the energy. This bandwidth is double the frequency deviation plus the maximum frequency of the input modulation content.
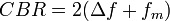
So to answer your question the spectral content is not necessarily directly related to the modulated signal spectra with FM. It's periodic, sure, but there is a lot of content. If you knew the exact modulation parameters you could try to work backwards and estimate the modulated input based on relative amplitudes and spectral frequencies.

