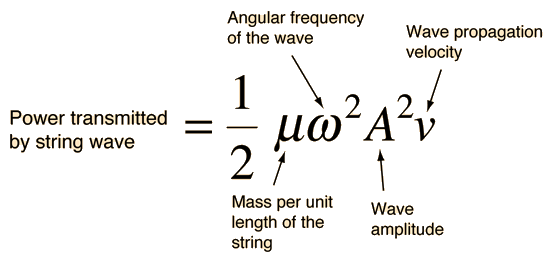Amplitude and angular frequencies are independent of one another. Take a look at position of vibrating spring.

$$x=Acos(\omega t)$$ Where $A$ is amplitude and $\omega$ is frequency. If you pull and release the spring after some moment, it will keep moving up and down. So it across the same after some moment. And graph of position of the spring will look like sinusoidal and cosinusoidal. And the bigger the amplitude is the bigger the phase will be.

Generally, angular frequency doesn’t care what amplitude.

Click on play button of $A$ in Desmos

Amplitude and angular frequencies are independent of one another. Take a look at position of vibrating spring.

$$x=Acos(\omega t)$$ Where $A$ is amplitude and $\omega$ is frequency. If you pull and release the spring after some moment, it will keep moving up and down. So it across the same after some moment. And graph of position of the spring will look like sinusoidal and cosinusoidal. And the bigger the amplitude is the bigger the phase will be.

Generally, angular frequency doesn’t care what amplitude.

Click on play button of $A$ in Desmos

From the answer, you can see that energy is proportional to 'angular frequency and amplitude' squared. $$E\propto \omega^2 A^2$$

As you increase or decrease the angular frequency the energy changes.

But if you want the energy to be unchanged then energy will be constant. Let's assume that E=1 J, $$1\propto \omega^2 A^2$$ $$\implies \frac{1}{\omega}\propto A$$. To keep the energy unchanged, if you change frequency then you have to change amplitude also. Since they are inversely proportional then if one increases then another will decreases.

Thanks to Cort Ammon

Amplitude and angular frequencies are independent of one another. Take a look at position of vibrating spring.

$$x=Acos(\omega t)$$ Where $A$ is amplitude and $\omega$ is frequency. If you pull and release the spring after some moment, it will keep moving up and down. So it across the same after some moment. And graph of position of the spring will look like sinusoidal and cosinusoidal. And the bigger the amplitude is the bigger the phase will be.

Generally, angular frequency doesn’t care what amplitude.

Click on play button of $A$ in Desmos

Amplitude and angular frequencies are independent of one another. Take a look at position of vibrating spring.

$$x=Acos(\omega t)$$ Where $A$ is amplitude and $\omega$ is frequency. If you pull and release the spring after some moment, it will keep moving up and down. So it across the same after some moment. And graph of position of the spring will look like sinusoidal and cosinusoidal. And the bigger the amplitude is the bigger the phase will be.

Generally, angular frequency doesn’t care what amplitude.

Click on play button of $A$ in Desmos