High energy orbitals can in some respects be represented by classical Bohr orbits but have strictly speaking still to be described my quantum mechanical wave functions, especially when it comes to calculating the transition probabilities to other states. High energy orbitals of all neutral atoms become increasingly hydrogen-like though for increasing n, which makes the transition probabilities easier to calculate. In any case, radiation is only emitted when the electron makes a transition to a lower level. This is a result of excited atomic states being quantum mechanically unstable (whether n is large or not), not because of the electron radiating as a classical particle. A classically radiating electron would continuously lose energy (producing a continuous spectrum over a wide frequency range in the process) and eventually spiral into the nucleus. This is obviously not observed. One only observes the discrete lines resulting from quantum mechanical transitions from level n to lower states.
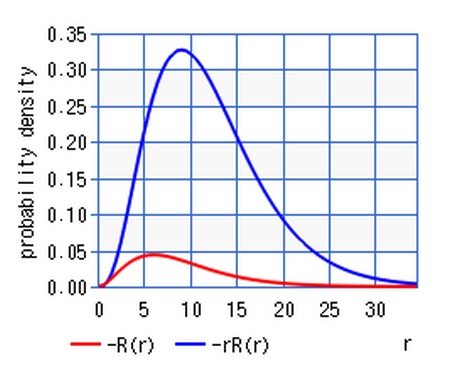
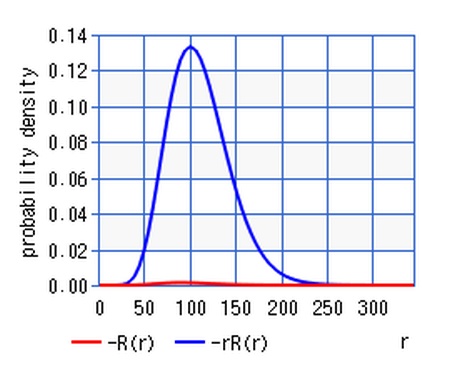
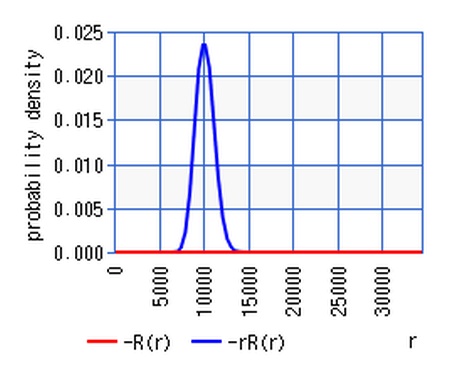
The plots below (produced at https://keisan.casio.com/exec/system/1224054805 ) show that, as mentioned above, the wave functions become relatively more sharply peaked with increasing n, but still are wave functions with a continuous spread and do not represent classical orbits.
n=100 (l=99)