Consider the expansion done for the kinetic energy of a system executing small oscillations as done in Goldstein:
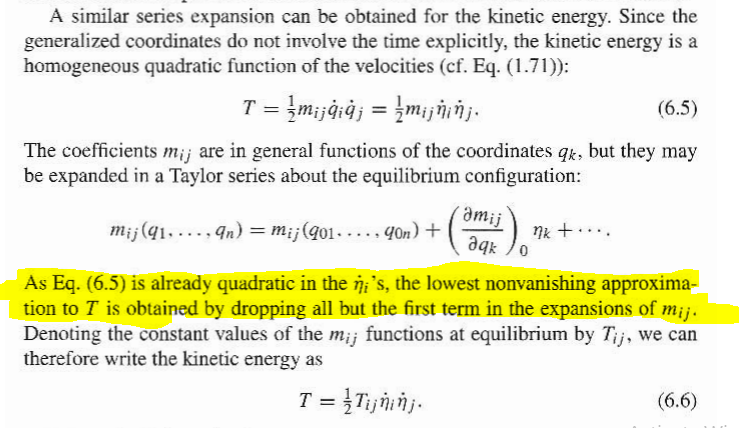
A similar series expansion can be obtained for the kinetic energy. Since the generalized coordinates do not involve the time explicitly, the kinetic energy is a homogeneous quadratic function of the velocities (cf. Eq. (1.71)): $$T=\frac{1}{2}m_{ij}\dot{q}_i\dot{q}_j=\frac{1} {2}m_{ij}\dot{\eta}_i\dot{\eta}_j\tag{6.5}$$ The coefficients $m_{ij}$ are in general functions of the coordinates $q_k$, but they may be expanded in a Taylor series about the equilibrium configuration: $$m_{ij}(q_1,...,q_n)=m_{ij}(q_{01},...,q_{0n})+\bigg(\frac{\partial m_{ij}}{\partial q_k}\bigg)_0 \eta_k+...$$ As 6.5 is already quadratic in the $\dot{\eta}_i$'s, the lowest nonvanishing approximation to $T$ is obtained by dropping all but the first term in the expansions of $m_{ij}$. Denoting the constant values of the $m_{ij}$ functions at equilibrium by $T_{ij}$, we can therefore write the kinetic energy as $$T=\frac{1}{2}T_{ij}\dot{\eta}_i\dot{\eta}_j\tag{6.6}$$
What does the highlighteditalicized part of the above quote mean?
How is the order of $\dot\eta$ the same as $\eta$?
As far as I know, if a function is small that doesn't guarantee that it's time derivative is also small.
Also, I have referred to many books and online lectures and none seem to explain this clearly.