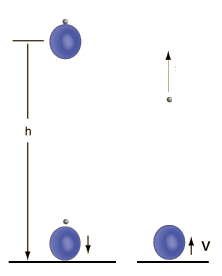The double ball drop problem is as follows:
A ball of mass $m$ is placed on top of a ball of mass $M$ (where $m < M$), and the balls are dropped simultaneously from some height $h$. When the balls hit the floor, the ball on top will shoot upwards. What is the velocity of this ball at the moment it shoots upwards?
And so I ask; is there any easy derivation of this problem, without the use of calculus?
I have attempted to solve it used the conservation of momentum, kinetic energy, and mechanical energy, but I seem unable to solve it. My attempt is as follows:
First, we can solve for the velocity of the larger ball using the conservation of momentum:
$$p_\text{before} = p_\text{after}$$
$$(m + M)\sqrt{2gh} = mv_m + Mv_M$$
$$v_M = \frac{(m + M)\sqrt{2gh} - mv_m}{M}$$
Then, using the conservation of kinetic energy:
$$\frac{1}{2}(m + M)\sqrt{2gh}^2 = \frac{1}{2}mv_m^2 + \frac{1}{2}Mv_M^2$$
$$v_M = \sqrt{\frac{2gh(m + M) - mv_m^2}{M}}$$
And then you can equate the two to get:
$$\sqrt{\frac{2gh(m + M) - mv_m^2}{M}} = \frac{(m + M)\sqrt{2gh} - mv_m}{M}$$
However, this, when solved, gives $v_m = \sqrt{2gh}$, which does not align with what I have been taught (saying that the velocity should be about three times this).
What am I doing wrong in this derivation? The math is correct, I'm sure. It's somewhere in the physics itself, a concept that I'm missing.
Any help is appreciated.