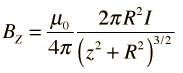I am trying to determine the external magnetic field of a solenoid at a given distance from its z$z$-axis. Currently, I have been able to find the biot savartBiot-Savart law for a current loop:
How $$B_{z}=\frac{\mu_{0}}{4\pi}\frac{2\pi R^{2}I}{(z^{2}+R^{2})^{3/2}}.$$ How would I determine the external magnetic field strength of a solenoid from this equation ?
Since each turn on a solenoid can be considered as one current loop, I presume there is a way to "add up" magnetic field strengths of a solenoid with n$n$ number of turns (current loops). We can also roughly equate the z squared + R squared , all to the power of 3/2,$(z^{2}+R^{2})^{3/2}$ as just z cubed$z^{3}$ , when z$z$ is significantly bigger than R$R$.
I think integrating the formula would give the answer, but since I am only familiar with very basic calculus ( ii.e. basic definite integrals), I am not sure how to go about this. If anyone uses calculus, please be so kind to add the steps for my own understanding (no matter how simple !)
(Note,I I only started 10th grade this fall, so please take this into account into your answers !)