I haven't fingured out how to completely solve the problem, but I have found two mistakes (of which I am absolutely certain of) in what you have done. Incorporating these two is still not giving the right answer, something else is wrong as well.
(1) You are using an incorrect expression for acceleration which is valid for classical cases only. That is, $\ a = \frac{\ v^2}{\ x}$ . The correct one is $\ a = \frac{\ v^2 \gamma}{\ x}$
These pages from Hartle's book Gravity will help. See example 5.6, equation 5.52 and whats written after it.
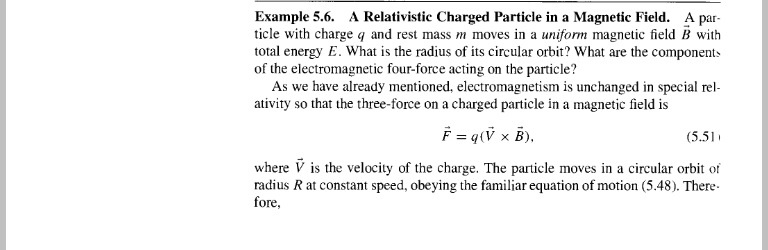
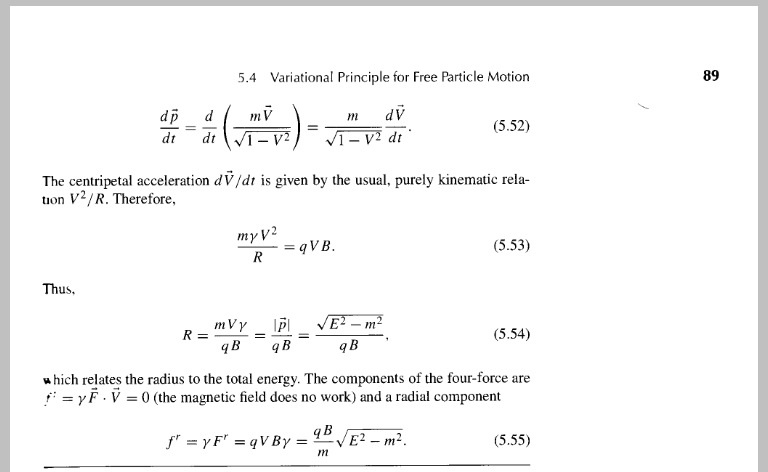
(2) $\ dt$ has to be corrected for. Use $\gamma\ {dt_{rest frame}}=d\tau_{rest frame}$ See the line following equation 11.119 in your textbook.
