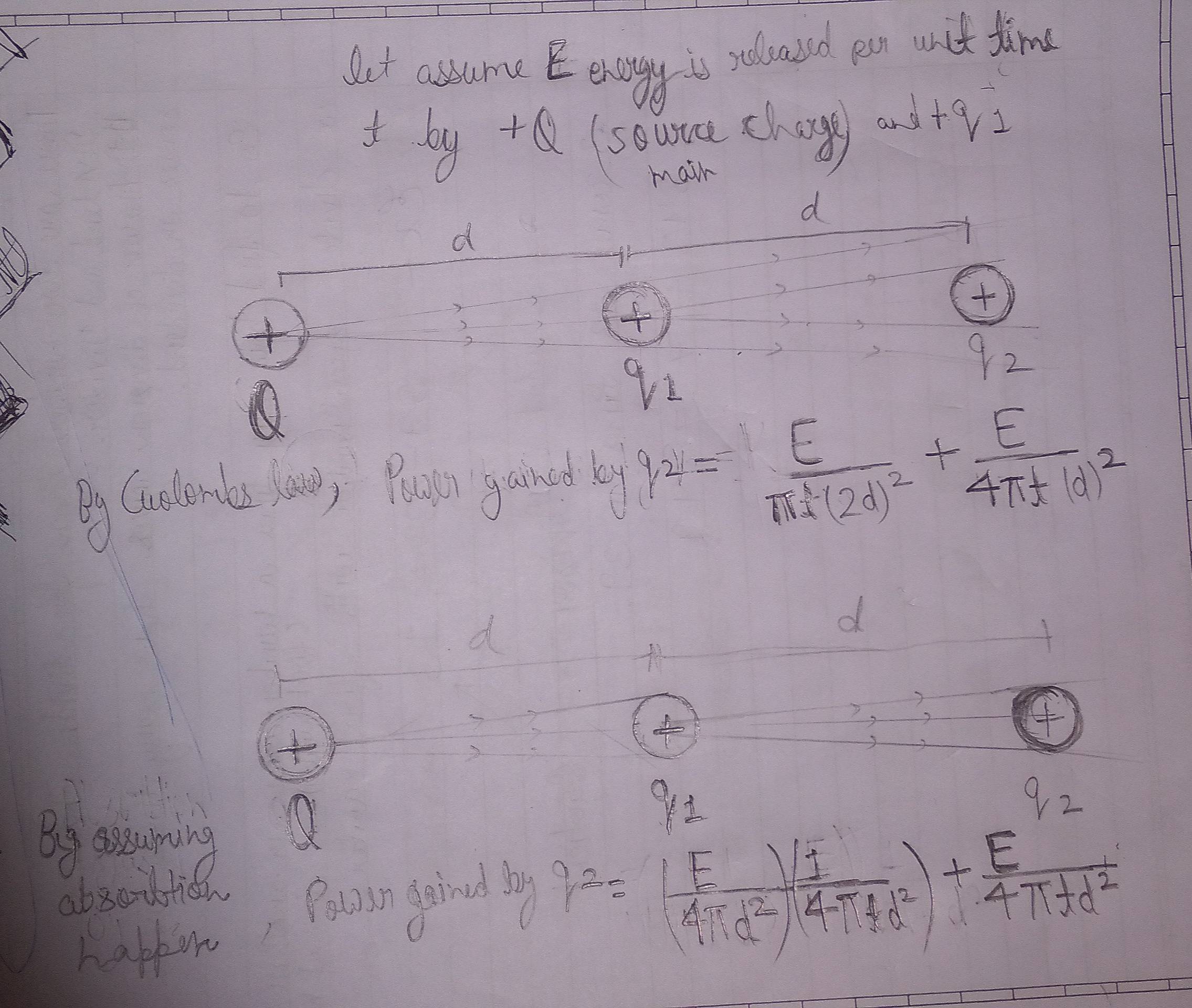
If we assume that energy was first absorbed by q1 and then released by it which then reaches q2 , the same as , electric field not being absorbable and somehow magically gives the energy to q2 and q1 with no reduction as seems by assuming that charge can absorb it. THE WHOLE SITUATION IS MADE TO BE STATIC
Energy gained by q2 in $t$ time $= (E/4(22/7)(2d)^2)+(E/4×(22/7)d^2)$
Which is different from assuming there is nothing like absorbtion, as it gives different value of energy gained by q2 in $t$ time $= (E/4(22/7)d^2)×1/4(22/7)d^2 + (E/4(22/7)d^2)$
EDIT: i think a more close assumption for that is , Electric fields do contain energy and can also pass through charges body and can also get absorbed by it But the absorption of that energy only happens when the field actually accelerate any charge(work done by producing motion) andOR charge can neither accumulate more or less energy density or electric field density. Take electric field strength(electric flux) proportional to Energy density