Despite your formula being wrong you get the correct result in this case. But all you did was calculate the electric field at one single point $\mathbf{r} = (0, 0, 0)^T$ which certainly doesn't say much about the field as a whole. The electric field of a single dipole $\mathbf{d}$ at $\mathbf{r}_0$ is given by
$$
\mathbf{E}(\mathbf{r}) = \frac{1}{4\pi\varepsilon_0}\frac{3(\mathbf{r}-\mathbf{r}_0) \mathbf{d}\cdot (\mathbf{r}-\mathbf{r}_0) - \mathbf{d} |\mathbf{r}-\mathbf{r}_0|^2}{|\mathbf{r}-\mathbf{r}_0|^5}
$$
If you redo your code like that an use a variable location at which to evaluate the electric field instead of choosing $(0,0,0)$ you will find a non zero field. It would look like this in the xy plane for example 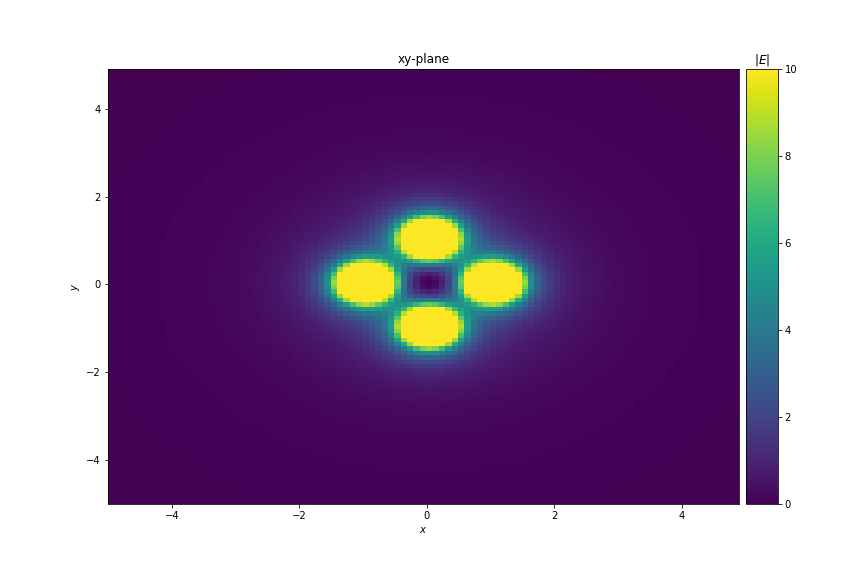
In the lecture Feynman is talking about an infinite chain along the z-axis of dipoles in the z direction and says that for a single dipole if you're a distance $r=z$ away from the dipole along the z-axis the field would be $$ \mathbf{E}(0,0,z) \propto \frac{3 z d_z z \mathbf{e}_z - d_z\mathbf{e}_z z^2}{z^5} = \frac{2z^2 d_z}{z^5}\mathbf{e}_z = \frac{2d}{z^3}\mathbf{e}_z $$ so the formula is not approximate, it's calculated for this special case. He then sums over the dipoles in this one chain and reasons that the other chains only contribute very little to the electric field.
