I think you are confusing which forces act on which object, but to be fair, I do not understand your solution at all. It seems to me to be wrong (but maybe I am just not understanding it).
You are asking about balance for inclined plane, therefore in your free body diagramthere needs to be only forces which act on the inclined plane in your free body diagram. I have no idea how did you get the forces you have there.
Your problem states that the inclined plane can move only horizontally, this means, that there is vertical force of constraint that compensates all vertical components of all other forces acting on the inclined plane. This force is missing from your free body diagram.
Next thing is that you do not wish to decompose the forces in the direction of the inclined plane. You are not interested in motion in this direction, you are interested in left-right motion, which is the only one allowed. Therefore, your condition is that the horizontal component of net force acting on the inclined plane should be zero (again, vertical component of net force is zero due to the force of constraint, which keeps the motion only horizontal, i.e. left-right)
Another thing is, that because the surfaces are frictionless, the only possible interaction between roller bearing and an inclined plane is by normal forces. Your $F_{s1}$ is however not normal to the inclined plane and thus this cannot be the force with which roller bearing acts on the inclined plane.
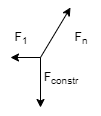
The free body diagram for an inclined plane should look like this (ignoring gravitational force acting on the inclined plane, but this is vertical so it is irrelevant for our problem. Let us say it is included in the force of constraint):
And the equation for vertical components is $$F_1=F_n\sin(\varphi)$$
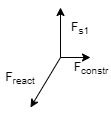
On the roller bearing we have this freebody diagram:
where $F_{react}$ is the reaction force to the normal force acting on the incline plane and $F_{constr}$ is again force of constraint, that keeps the string vertical. Thus: $$F_{s1}=F_{react}\cos(\varphi)=F_n \cos(\varphi)$$ And combining the equations we get $$F_{s1}=\frac{F_1}{\tan\varphi}.$$