The equation for Thermal Resistancethermal resistance R is:
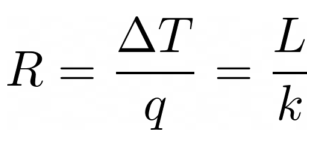 $$R=\frac{\Delta T}{q}=\frac{L}{k}$$
$$R=\frac{\Delta T}{q}=\frac{L}{k}$$
with
- T being the temperature difference (in Kelvinkelvins)
- q being the Heatheat flow rate (in W/m2m²)
- L being the thickness of the material (in metres)
- k being the Thermalthermal conductivity (in W/(mK) Watts, watts per metre kelvin)
Whether you use temperature and q, kelvin / (Watts x metres^2) => (metres^2 x Kelvin) / Watts or L and k, metres / (Watts / (metre x kelvin)) => (metres^2 x Kelvin) / Watts:
temperature and q, kelvin / (watts × metres²) ⇒ (metres² × kelvin) / watts or
L and k, metres / (watts / (metre × kelvin)) ⇒ (metres² × kelvin) / watts,
they both give you obtain (metres^2 x Kelvinmetres² × kelvin) / Wattswatts. This makes sense, as (m^2 xm² × K) / W is the units for thermal resistance.
So why do datasheets for electronic components give thermal resistance aswith units of °C/W? I understand °C/W likely means for every Wattwatt of power wasteddissipated by the device, the device will riseheats up by that temperature. But how can itthis parameter have two different units?
Is 8°C/W the same as 8 (m^2 xm² × K) / W?
