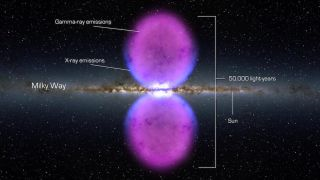
Observations of galaxies commonly show ‘jets’ and other ejection phenomenon like these ‘Fermi bubbles’
"The Fermi Bubbles are two enormous orbs of gas and cosmic rays that tower over the Milky Way, covering a region roughly as large as the galaxy itself. These giant space bubbles may be fueled by a strong outflow of matter from the center of the Milky Way."
The Fermi Bubbles are two enormous orbs of gas and cosmic rays that tower over the Milky Way, covering a region roughly as large as the galaxy itself. These giant space bubbles may be fueled by a strong outflow of matter from the center of the Milky Way.
Source: Something Strange Is Happening... https://www.space.com/fermi-bubbles-milky-way-radiation-mystery.htmlSomething Strange Is Happening in the Fermi Bubbles (Space.com)
Even the Big Bang is thought by some to be a ‘Big Bounce’. To explain these things, it seems desirable to have the feature, in a theory of gravity, that the strength of gravity reduces for dense regions of matter.
It would also help avoid infinity problems, such as those predicted by General Relativity in the singularity of a black hole.
But does General Relativity allow such a feature? Can it be interpreted or amended to allow it?
Apparently, mass and energy increase the curvature of space-time (gravity) and so does pressure, so that, for example, at the center of a galaxy, the pressure would add to the strength of gravity and not reduce it.
But using a Newtonian approach, a reduction is predicted and here is the work done so far.
I’m wondering whether General Relativity can be interpreted in such a way as to reach a similar conclusion.
For a universe near critical density, for each mass $m$
$mc^2-\frac{GMm}{R^2} = 0$$$mc^2-\frac{GMm}{R^2} = 0$$
or rearranging to make $G$ the subject
$G=\frac{Rc^2}{M}$$$G=\frac{Rc^2}{M}$$
Small numerical constants omitted, $M$ is the mass of the visible universe within radius $R=\frac{c}{H}$ and $H$ is Hubbles constant.
Why it’s true is similar to the ‘flatness problem’.
If we just accept it, and accept it’s true for any mass, then for a larger mass, or one with a small radius $r$, and we include the ‘self gravitational energy’
$mc^2-\frac{GMm}{R^2} - \frac{Gm^2}{r} = 0$$$mc^2-\frac{GMm}{R^2} - \frac{Gm^2}{r} = 0$$
it rearranges to
$G_{effective}=\frac{c^2}{c^2/G + m/r}$$$G_{effective}=\frac{c^2}{c^2/G + m/r}$$
and a reduction of $G$ is predicted for dense matter.
Is there any way that General Relativity can be interpreted to allow a reduction, not an increase, of the strength of gravity for dense regions of matter?