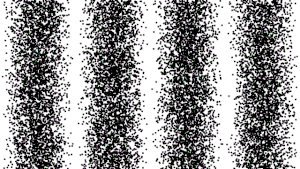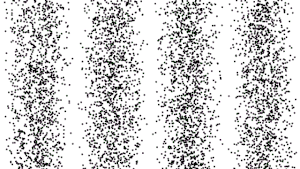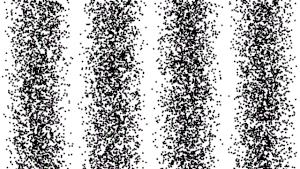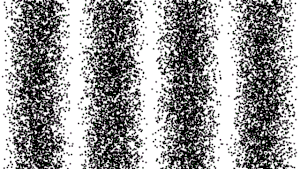What is a wave function? It is a mathematical function depending on energy and momentum or space and time,$Ψ(p_x,p_y,p_z)$ or $Ψ(x,y,z,t)$ ( in its simple form). This function is a solution of a wave equation, a second order differential equation.

Mathematical functions are a billion, what is the wave function's connection with measurable physical quantities? The connection is postulated axiomatically in the postulates of quantum mechanics¨, $Ψ^*Ψ$ is the probability distribution of the products of the interaction; this means that a number of measurements have to be done with the exact boundary conditions to get an experimental distribution to compare with the theory that has calculated the wavefunction. So the mathematical function itself is not directly attributed to a given event, so it cannot be measurable.

How is a measurement performed?by interaction. Each interaction changes the boundary conditions, and thus the specific mathematical $Ψ$ is different before or after the measurement. That is what the collapse is, change of the specific wavefunction.

I will continue

It says that for a given boundary condition physical problem the

What is a wave function? It is a mathematical function depending on energy and momentum or space and time,$Ψ(p_x,p_y,p_z)$ or $Ψ(x,y,z,t)$ ( in its simple form). This function is a solution of a wave equation, a second order differential equation.

Mathematical functions are a billion, what is the wave function's connection with measurable physical quantities? The connection is postulated axiomatically in the postulates of quantum mechanics¨, $Ψ^*Ψ$ is the probability distribution of the products of the interaction; this means that a number of measurements have to be done with the exact boundary conditions to get an experimental distribution to compare with the theory that has calculated the wavefunction. So the mathematical function itself is not directly attributed to a given event, so it cannot be measurable.

How is a measurement performed?by interaction. Each interaction changes the boundary conditions, and thus the specific mathematical $Ψ$ is different before or after the measurement. That is what the collapse is, change of the specific wavefunction.

You state:

the wave function collapses immediately everywhere.

If one has to choose a different wavefunction, since it is a mathematical construct of course it can change immediately everywhere.

I have found it useful in understanding the difference between probability distributions and the need for a different wavefunction, to contemplate the single electron double slit experiment.

Each little dot seems random, but it is one materialization of the "collapse of the wavefunction" (btw I think the term collapse is not very smart, the wavefunction is not a balloon).

Before the electron hits the screen it has one $Ψ$, after it hits the screen it has another given by new boundary conditions:electron interacting with atoms in the screen, a completely different function.

Does it take time for the transform? Of course as the interaction is electromagnetic , nothing is "immediate" in that sense, it takes time to change experimental boundary conditions. Note though that the result of one electron is a single dot, not a function in space and time all over the place. The probability distribution responsible for the accumulation of many many dots, is bounded by the boundary conditions of the original problem "electron scattering off two slits given distance apart , given width".