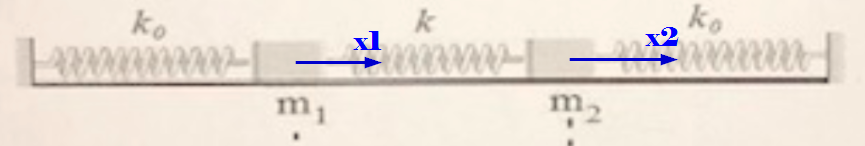
I suggest stick to convention and do the free body diagram with positive displacements towards the right.
In fact I drew the above with $x_2 > x_1$ to help with the determination of the middle spring force direction.
From the above I have
$$ \begin{aligned} F_1 & = k_0 (x_1) \\ F_2 & = k (x_2-x_1) \\ F_3 & = k_0 (x_2) \end{aligned} $$
and the equations of motion
$$ \begin{aligned} F_2 - F_1 & = m_1 \ddot{x}_1 \\ -F_2 - F_3 & = m_2 \ddot{x}_2 \end{aligned} $$
Combined the above produces
$$\begin{bmatrix} m_1 & 0 \\ 0 & m_1 \end{bmatrix} \pmatrix{\ddot{x}_1 \\ \ddot{x}_2 } = \begin{bmatrix} k+k_0 & -k \\ -k & k+k_0 \end{bmatrix} \pmatrix{x_1 \\ x_2} = \pmatrix{0\\0} $$$$\begin{bmatrix} m_1 & 0 \\ 0 & m_1 \end{bmatrix} \pmatrix{\ddot{x}_1 \\ \ddot{x}_2 } = -\begin{bmatrix} k+k_0 & -k \\ -k & k+k_0 \end{bmatrix} \pmatrix{x_1 \\ x_2} $$
Now you can go and flip the sign of $x_1$ and of $\ddot{x}_1$ if you want.