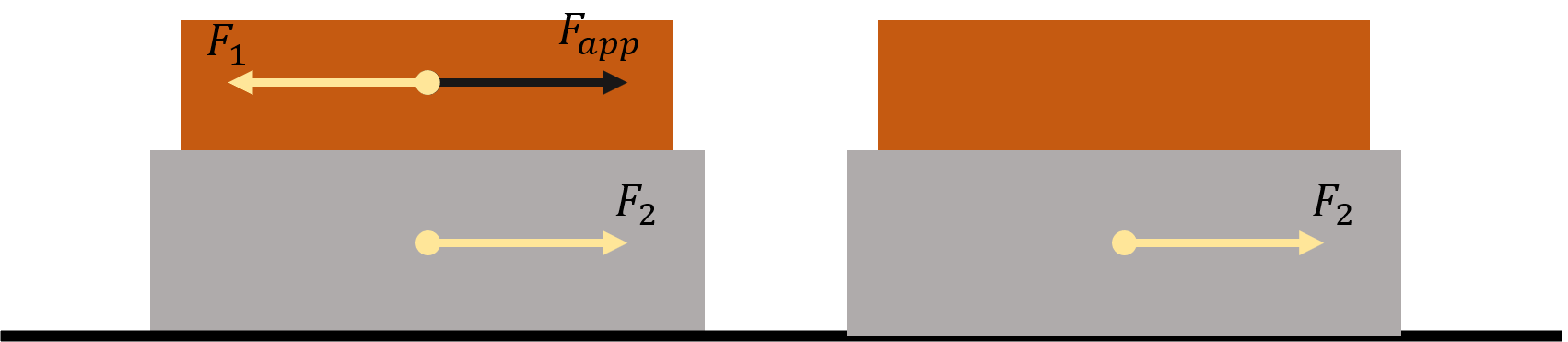After having attended a specific mechanics course I've struggled with a certain problem, which is related to static friction. First of all, if some force is applied to a block (the source of the applied force is not of importance in this scenario), that's placed on top of another block, it's going to accelerate in case there's no static friction to cancel the applied force. However, as the left picture below suggests, there's a static frictional force between the two blocks, and for simplicity no frictional force between the lower block and the ground. Now the static friction will always be equal in magnitude to the applied force but in the opposite direction, at least to a certain level. As can be seen, $F_1$ compensates for $F_{app}$, at the same time, Newton's third law states that each applied force has an equal and opposite force which acts on the other object. Hence, the static frictional force $F_2$ is applied on the lower block, so I would say that the net force on the above block will become 0N and the lower block will experience a net force of $F_2$. As a result the above block will remain stationary and the lower block is going to accelerate, but this final result doesn't seem to make sense to me: the upper block remaining still why the lower block is going to accelerate. I must miss some crucial point about the static frictional force, but what? Thanks.