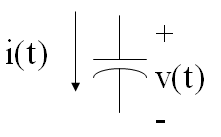You don’t change the sign because the capacitor is discharging. The fundamental equation presupposes the current flowing into the capacitor (as you have it in your drawing). The negative sign will take care of itself in the solution (discharging).
$$ C \frac{dV_c(t)}{dt} = -\frac{1}{4k} V_c(t) $$
The fundamental capacitor equation being, $$i(t)=C\frac{dv}{dt}$$ If
If you want to define your current as leaving the capacitor then you need to include a negative sign in your dv/dt term.