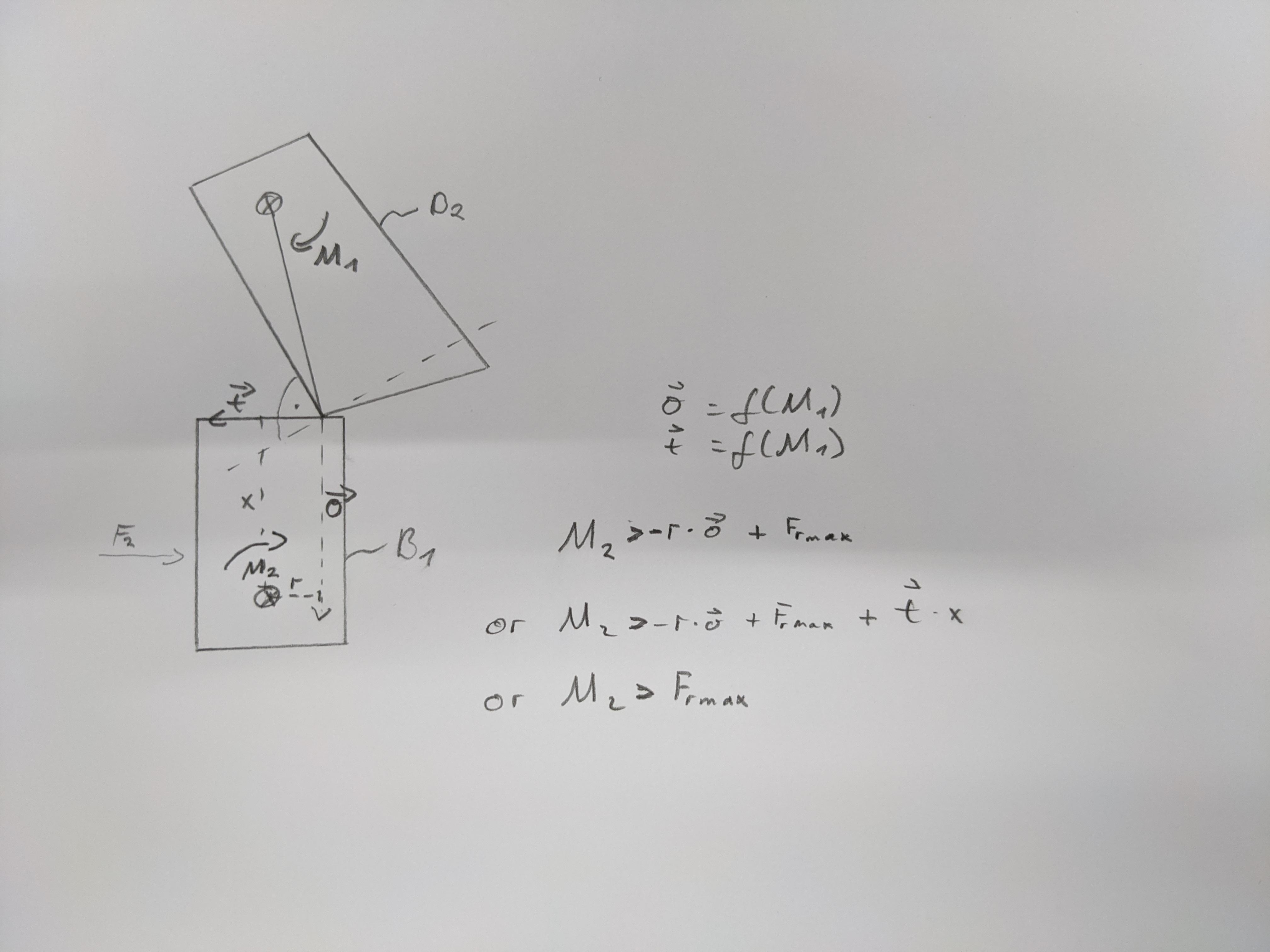
Suppose two bodies B1 and B2 are in contact with each other in a static situation. A torque M1 is applied to the first body B1B2. The torque M1 is transmitted to the second body B2B1. Both bodies won't move because they hinder each others movement due to the inclined contact surfaces.
Now my first question is, what force is transmitted from B1 to B2 to B1 directly originating from M1. More particular, in which direction does the force point?
My guess is that the force points orthogonally away from the contact contour of B2 (dotted line). This force would the be split into a transversal component t and an orthogonal component o. In this case, t is always samllersmaller then or equal to the maximum static friction (t <= normal force o * (static friction coefficient), meaning t <= Frmax = o * mu).
Alternatively, the force could point orthogonally away from the surface of B1, which would then have only a component in direction o with t = 0.
Another alternative, which I think I have excluded, is that the force points in a direction tangential to the movement path of the contact point, which is circular around the pivot axis of B2.
The second question is, how big does a second torque M2 have to be to be able to move the second body B1 into clockwise direction (M2 results from F2, but lets ignore the lever arm).
I have three possible equations. Basically the question is, can the force t be transmitted by friction and work against M2, making it necessary to overcome t (muliplied with the lever arm x to get a torque)? I could not figure out if static friction is overcome by the difference of forces (M2 - r * o) or the maximum of forces (max(M2,r + o)). (I think Frmax is technically defined incorectly because there is also a lever arm between M2 and Frmax, but if that one doesn't matter in principle, lets ignore that)
The first two equations are based on the assumption that the normal part of the transmitted force o mulitplied by the lever arm r will be added to M2, making the necessary M2 less big. Or is that assumption wrong, making the third equation correct?
P.s. This question losely expands my previous question.