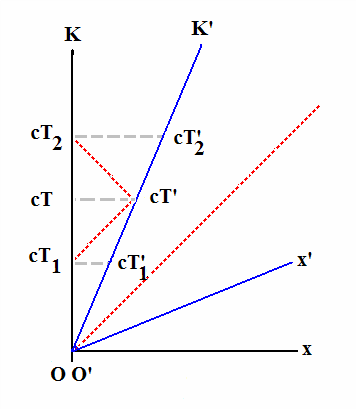
I am currently studying special relativity mainly using this paper which uses spacetime diagrams to derive the formulas for the properties of special relativity (Time dilation, length contraction etc.).
In chapter II, the "Doppler k-Factor" is derived as $$k=\sqrt{\frac{1+\beta}{1-\beta}} \tag{9}$$ where $\beta=\frac{V}{c}$ ($V$ is the speed of which somean inertial frame of reference $K'$ is receding from a "stationary" frame $K$.
(I use the same tags for equations here as used in the linked paper to avoid confusion).
Earlier in the paper, $k$ has been defined as $$k=\frac{cT_2}{cT'}=\frac{cT'}{cT_1}\tag{7}$$
You can get from here to euquation $9$ by multiplying the two "versions" of $k$ and then taking the square root.
$cT_2$, $cT_1$ and $cT'$ are different "events" that happen when a ligght ray is sent from $K$ to $K'$ and reflected from $K'$ back to $K$:
(illustrationIllustration from the linked paper)
Where it gets confusing for me is in chapter IIIII,I where the doppler factorDoppler Factor is used to derive the formula for time dilation. It is said that
$$\frac{cT_2}{cT'}=\frac{(1+\beta)cT}{cT'}=\frac{1}{k}\tag{10}$$
The first step makes sense since $cT_2$ is simply replaced by $(1+\beta)cT$ which havehas been derivedshown to be the same earlier:
$$cT_2=cT+VT=(1+\beta)cT\tag{4}$$
But how is $\frac{cT_2}{cT'}$ equal to $\frac{1}{k}$? As we saw in equation $7$, $k=\frac{cT_2}{cT'}$, which means that $$k=\frac{1}{k}$$
Obviously, this cannot be true. So what am I missing here? How does one get to $\frac{cT_2}{cT'}=\frac{1}{k}$?
Please note that I (unfortunately) do not have physics class in school, so I cannot ask any teacher. I apologize if the question/answer is trivial.