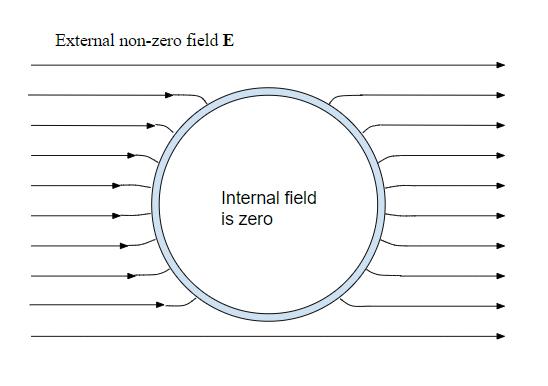
In many introductory books on electrostatics, you can find the statement that the field inside a conducting shell is zero if there are no charges within the shell. For example, if we place an uncharged conducting sphere in a uniform electric field we would get something like this:
For simplicity, I have drawn a uniform external field and a spherical shell, but the internal field is zero for any external field and any shape of the shell.
We know the field in the metal of the conductor is zero, so the external field must induce a charge separation in the conductor and this charge separation produces a field that cancels out the external field within the metal of the shell (shaded blue in the diagram). However, there is no obvious reason why the field should also be canceled to zero in the interior of the shell.
Although it is widely stated in introductions to electrostatics that the field is zero in the interior of the shell I cannot find proof of this. So the questionquestion is how we prove that field inside the shell is always zero whatever the external field and shape of the shell?