Slip is not "impending in the direction in which the wheel rolls". Slip is impending in the opposite direction to which which the present forces are pushing it.
And the force to consider here is gravity; it is trying to make the contact point slip by pulling the ball downwards, so static friction must pull upwards to prevent the contact point from slipping.
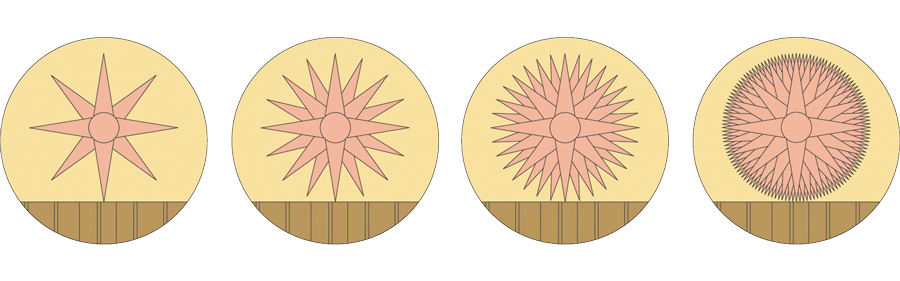
A fruitful way of thinking about this is by imaging a star rolling down the inlince. (On this image the star is on flat ground, but imagine the ground being tilted.)
To have "rolling" without slipping, each leg must not slip while in contact with the incline surface. Gravity pulls downwards, so static friction must pull upwards to avoid that the leg slides down.
With more legs, the same is still the case. Each leg takes over right as the previous leg let's go, but while it is in contact it mustn't slide. Gravity causes this sliding, so static friction must point upwards.
With even more legs, the same is still the case.
With so many legs that we basically have a continuous circular surface - with infinitely many legs that are infinitely close but also infinitely small, so just one point each. Each point is still a leg, so for this wheel, the above description still counts while a point is touching the surface: Gravity pulls down trying to make it slide, so static friction must pull up to prevent it.