TL;DR: Tree-level flavour changing neutral currents are not allowed in the Standard Model. There could be, if you invent another particle or two (and indeed there are New Physics proposals about this).
But the Standard model does allow for flavour changing neutral currents with higher order diagrams, like box or penguin diagrams.
More in depth
A "flavour changing current" is a process featuring the violation of quark flavour conservation. When mediated by a neutral particle such as the $Z^0$ boson (or a photon), they do not entail a net charge exchange and are hence called "flavour changing neutral currents".
if a top quark emits a Z boson or a Higgs boson
At tree level the $Z^0$ boson cannot allow flavour mixing.
This is because the $Z^0$ boson has no charge and can hence only couple quarks of the same charge: so both $i$ and $j$ have to be $\in (u, c, t)$ or $\in (d, s, b)^\dagger$. You can show, then, the matrix element for the tree level interaction goes as : $$ \mathcal{M} \quad \propto \quad g_W \cdot(u, c, t) \left (\begin{array}{c} \bar u \\ \bar c \\ \bar t \end{array} \right ) \quad \propto \quad u\bar u + c \bar c + d \bar d,$$ i.e. no cross-generational coupling and hence no flavour mixing.
If the process were mediated by a $W^{\pm}$ boson, on the other hand, you'd have sometimesomething like:
$$ \mathcal{M} \quad \propto \quad \bar u\bar V_{ud} d + \bar u V_{us} s + ...,$$
i.e. cross-generational coupling and hence flavour mixing.
$V$ is the CKM matrix.
Hence, the lowest order diagrams for Flavour Changing Neutral Currents in the Standard Model are box or penguin diagrams:
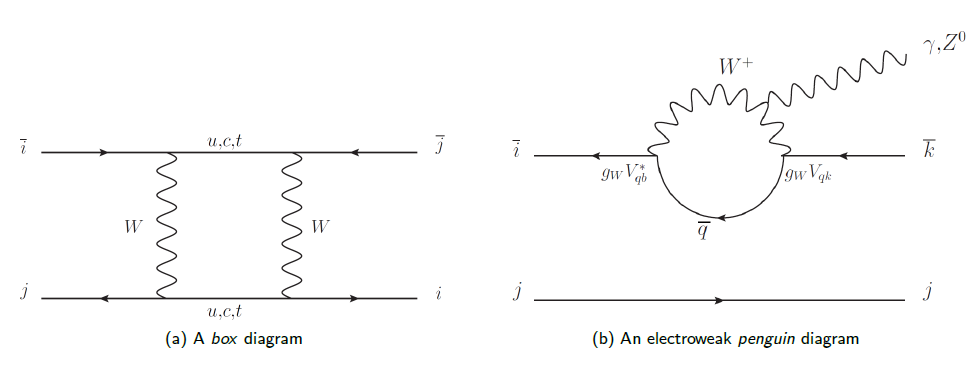
The penguin diagram gets its name because:
$\dagger$: There are some subtleties about the mass eigenstates $(d,s,b)$ not being the same eigenstates of the weak force $(d',s',b')$. The two bases are related by the CKM matrix. I ignore all of this, just to provide a qualitative picture.