Does it make sense to have a spacetime in general relativity that has a finite extent or boundary.
Yes. It could be done and makes a lot of sense in some cases.
For example we could start with a manifold without a boundary that has a discrete symmetry group acting on it with a fundamental domain which is a manifold with boundary consisting of fixed points of this symmetry group. We could then consider a quotient space of manifold by this symmetry as a manifold with boundary (this is an example of orbifold construction). Gravity theory living on original full manifold will also have an equivalent description as a theory on a quotient manifold with boundary. People refer to these two viewpoints as “upstairs” (full manifold without boundary) and “downstairs” descriptions. Sometimes this downstairs description offers additional insight into physics.
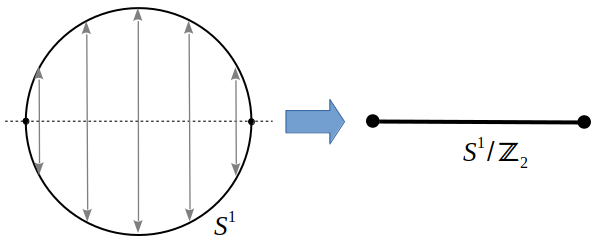
An important example is Hořava–Witten theory: the low energy limit of 11 dimensional M-theory defined on $M_{10}\times S^1/\mathbb{Z}_2$ is equivalent to strong coupling limit of heterotic $E_8 \times E_8 $ string theory (see original works paper 1, paper 2, or these lectures). The $\mathbb{Z}_2$ acts on a circle $S^1$ as a reflection by a diameter. So the $ S^1/\mathbb{Z}_2$ is equivalent to a line segment with two endpoints (see figure):
Conceptually what does it even mean to have a spacetime with boundaries?
It means that in addition to the bulk gravitational theory there is also the dynamics of the boundary: a field theory on a codimension 1 manifold. In the Hořava–Witten example there is no Yang–Mills supermultiplet in the 11 dimensions, but each of the two 10-dimensional boundaries (corresponding to two endpoints of line segment) has a super Yang–Mills field theory with adjoint $E_8$ “living” in it. And so the boundary emerges as an important dynamical entity, a brane (in H–W model it would be called an M9–brane, reflecting its M–theory origin and its 9 spatial dimensions).
How does this change in a model of quantum gravity (at least the current avenues of study)?
Hořava–Witten theory is an important example of superstring/M-theory dualities, so this is an example of quantum gravity model. One of the insights gained from these examples is that dynamics of extended objects (branes) including their world-volume field theories is an important ingredient of quantum gravity models (at least those that originated from string theory).