From Maxwell's equations you use the many derivations to get Poynting's theorem: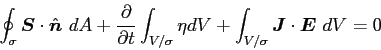
Since the fields are static, the second term is zero. The third termrate at which mechanical work is also zerodone on each charge is $e\mathbf E \cdot \mathbf V= \mathbf E\cdot \mathbf J$. This needs to ensurebe zero so that the $\vec J$ remains constant$\mathbf {J}$s don't change, keeping $\mathbf B$ static. So you're left with your expression equaling zero.
