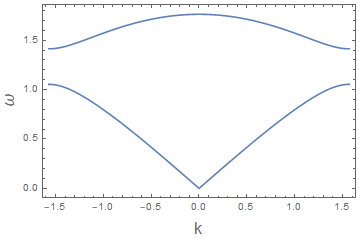
This is the way I like to think about it. You have probably encountered dispersion relations already, which tell you the frequency for a particular wavelength. Here's the dispersion for phonons (vibrations in crystals):
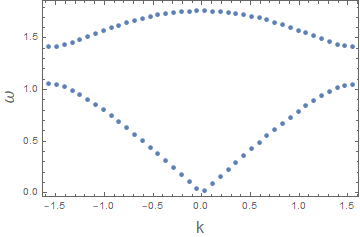
In quantum mechanics frequency and wavelength are related to energy and momentum respectively by $E=\hbar\omega$ and $p=\hbar k$. Each material has its own dispersion relation and this dispersion places constraints on the allowed energies. A free particle has dispersion $E=\hbar^2 k^2/2m$ so any energy is possible but take for example the infinite square well. There the potential forces the energies to only take on discrete values. To go back to the phonon dispersion, let's break up the dispersion so it only takes on discrete values of $k$ to make it easier to visiualize.
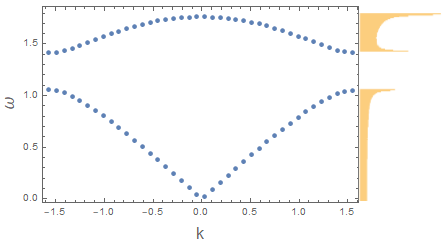
Now imagine you turn the image $90^\circ$ clockwise and let all the points fall down. If you now count how many points fell onto a particular energy you roughly see how many states are available for that energy level. This looks something like this:
For energy bands we are only interested in whether or not an energy has any available states. To get the energy band you extend the yellow graph so that it reaches all the way if has at least one state state. You basically stretch the yellow graph until it is a rectangle. For some energies there are zero states so even after this stretching it is still empty. This is a band gap.
In quantum mechanics each state can only be occupied by one particle (for fermions, which include electrons) so usually the states are filled from the bottom up. Energy and momentum conservation still apply so to excite an electron from the lower to the upper band it has to absorb an energy that is at least as large as the band gap.
Edit in response to @garyp: I used energy dispersion a bit loosely. Phonons do have a dispersion and are a nice example but this 'band gap' is different from that in semiconductors. Phonons are not quantum objects so the frequency of a phonon is not its energy. To get electronic dispersion relationships like for semiconductors you would have to solve the Schrödinger equation and then the resulting dispersion will give you the energy.