At the introduction to quantum mechanicmechanics, phase $v_p$ and group $v_g$ velocities are often presented. I know how to derive $v_p$ and get the equation:
$$ \scriptsize v_p=\frac{\omega}{k}. $$
What i dontI don't know is how to explain a derivation of a group velocity $v_g$ to myself. Our professor did derive it, but iI am having some difficulties with it.
1stFirst, he did a superposition of 2 waves with the same amplitude $s_0$:
$$ \scriptsize \begin{split} s &= s_0 \sin(\omega_1t-k_1x) + s_0 \sin(\omega_2t - k_2 x)\\ s &= s_0 \left[ \sin(\omega_1t-k_1x) + \sin(\omega_2t - k_2 x) \right]\\ s &= 2s_0 \left[ \sin\left(\frac{(\omega_1t-k_1x)+(\omega_2 t -k_2 x)}{2}\right) \cdot \cos\left(\frac{(\omega_1t-k_1x)-(\omega_2t - k_2 x)}{2}\right) \right]\\ s &= 2s_0 \left[ \sin\left(\frac{\omega_1 + \omega_2}{2} t - \frac{k_1 + k_2}{2} x\right) \cdot \cos\left(\frac{\omega_1 - \omega_2}{2} t - \frac{k_1 - k_2}{2} x\right) \right]\\ s &= 2s_0 \left[ \sin\left(\overline \omega t - \overline{k} x\right) \cdot \cos\left(\frac{\Delta \omega}{2} t - \frac{\Delta k}{2} x\right) \right]\\ \end{split} $$
Here, $\overline \omega$ is larger than $\Delta \omega$ and this is why:
- $\scriptsize\sin \left(\overline{\omega}t - \overline k x\right)$ is athe part which declares an envelope and
- $\scriptsize\cos \left(\frac{\Delta{\omega}}{2}t - \frac{\Delta k}{2} x\right)$ is athe part which declares phases inside an envelope.
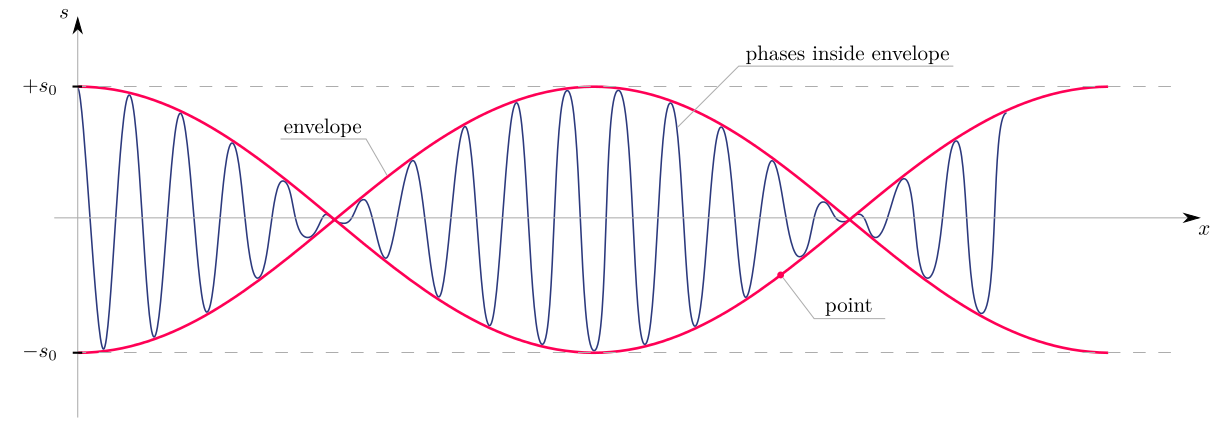
ThanThen, the professor takes only athe part which declares an envelopaenvelope and says that the phase of this part must be constant, like this:
$$ \scriptsize\frac{\Delta{\omega}}{2}t - \frac{\Delta k}{2} x = const. $$
QUESTION: What does this mean? Does a constant phase mean to only look at one point which is allwaysalways at the same distance from the $x$ axis? Please someone explain this a bit.
WellThe professor then he derivesgoes on to derive the group velocity easily from now on like this:
$$ \scriptsize \begin{split} \frac{\Delta{\omega}}{2}t - \frac{\Delta k}{2} x &= const.\\ \frac{\Delta k}{2} x &= \frac{\Delta{\omega}}{2}t - const.\\ x &= \frac{\Delta{\omega}}{ \Delta k} t - \frac{2}{\Delta k}const.\\ \end{split} $$
If iI partially diferentiatedifferentiate $x$ i, I finally get the group velocity:
$$ \scriptsize \begin{split} v_g &= \frac{\partial x}{\partial t} \\ v_g&= \frac{\Delta{\omega}}{ \Delta k}\\ v_g&= \frac{\textrm d{\omega}}{ \textrm d k} \end{split} $$
