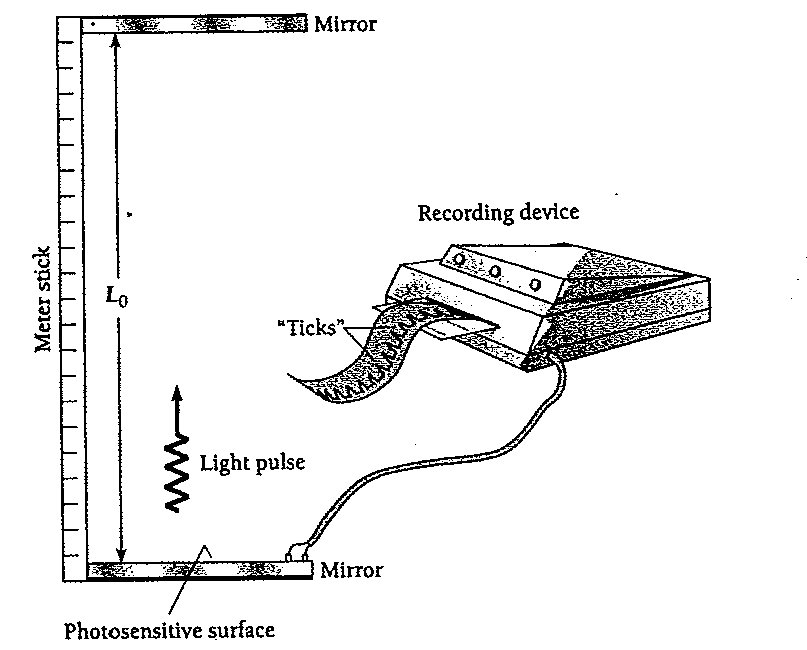
If you are just curious as to how they came up to the value: $$\sqrt{1-\frac{v^{2}}{c^{2}}}$$ The basis of the calculation is on time dilation.
The time interval between ticks is the proper time $t_0$ and the time needed for the light pulse to travel between the mirrors at speed of light $c$ is $\frac{t_0}{2}$. Hence, $\frac{t_0}{2} = \frac{L_0}{c}$ and $$t_0 = \frac{2L_0}{c}$$
The time interval between ticks is $t$. Because the clock is moving, the light pulse, as seen from the ground follows a zigzag path. On its way from the lower mirror to the upper one in the time $\frac{t}{2}$, the pulse travels a horizontal distance of $v(\frac{5}{2})$$v(\frac{t}{2})$ and a total distance of $c(\frac{t}{2})$. Since $L_0$ is the vertical distance between mirrors,
$$(\frac{ct}{2})^2 = L_0^2 + (\frac{vt}{2})^2$$ $$(\frac{t^2}{4})(c^2 - v^2) = L_0^2$$ $$t^2 = \frac{4L_0^2}{c^2 - v^2} = \frac{(2L_0^2)}{c^2(1 - \frac{v^2}{c^2})}$$ $$t = \frac{\frac{2L_0}{c}}{\sqrt{1-\frac{v^2}{c^2}}}$$
Time dilation now is
$$t = \frac{t_0}{\sqrt{1-\frac{v^2}{c^2}}}$$
Thus,
$$\sqrt{1-\frac{v^2}{c^2}} = \frac{t_0}{t}$$
Where:
$t_0$ = time interval on clock at rest relative to an observer = proper time
$t$ = time interval on clock in motion relative to an observer
$v$ = speed of relative motion
$c$ = speed of light