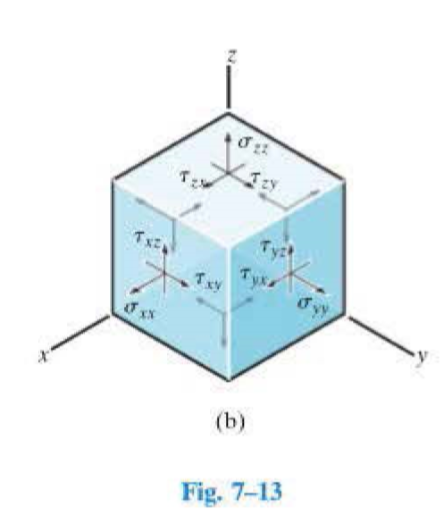
I am trying to understand the derivation of the following equation which describes the motion of Newtonian viscous fluids:
Equation 1: $\rho \dfrac{D u}{Dt} = \rho g_x - \dfrac{\partial p}{\partial x} + \mu \nabla^2 u$
Where $\mu$ is the viscosity of the fluid. I am following the proof written in Hibbeler's Fluid Dynamics which uses the following steps.
First the following formula is stated based on the free-body diagram below: (I am just writing the equations related to the $x$ component of the velocity, namely $u$)
Equation 2: $\rho \dfrac{D u}{Dt} = \rho g_x + \dfrac{\partial \sigma_{xx}}{\partial x} + \dfrac{\partial \tau_{yx}}{\partial y} + \dfrac{\partial \tau_{zx}}{\partial z}$
In the second step, the normal stress and shear stress variables in the previous equation are related to the velocity and viscosity of the fluid.
$\sigma_{xx} = -p + 2\mu \dfrac{\partial u}{\partial x}$
$\tau_{yx} = \mu (\dfrac{\partial u}{\partial y} + \dfrac{\partial v}{\partial x})$
$\tau_{zx} = \mu (\dfrac{\partial u}{\partial z} + \dfrac{\partial w}{\partial x})$
Here the book claims that by replacing the last three equations in equation(2), we yield equation(1).
I do not understand how the last three equations are derived. Moreover, after replacing these last three equations in equation(2), the result is not even similar to equation(1). This is what I got after doing so:
$\dfrac{Du}{Dt} = \rho g_x - \dfrac{\partial p}{\partial x} + \mu (\nabla^2 u + \dfrac{\partial^2 u}{\partial^2 x} + \dfrac{\partial^2 v}{\partial x \partial y} + \dfrac{\partial^2 w}{\partial x \partial z})$
What am I doing wrong? Is it possible the three equations presented in step 2 are wrong? Is there a source which explicitly explains this topic?
Thanks for your help!