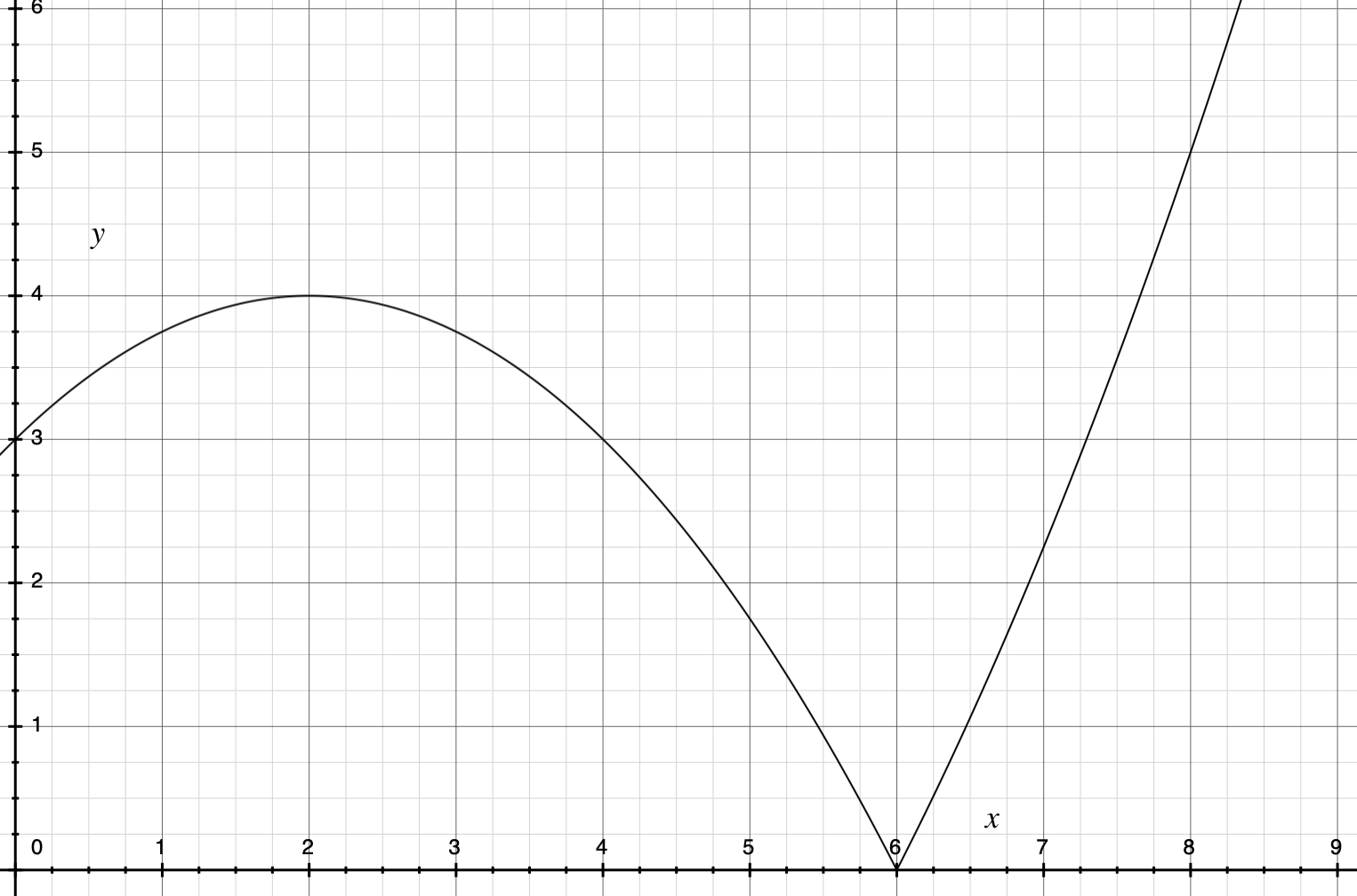
The tricky part of this question is that you are given a graph of velocity but asked a question about speed.
Several others have said essentially the same thing, but what really makes this clear for me is a graph of speed:
The above is the graph of $$ y = \left \lvert 4 - \left ( \frac{x - 2}{2} \right ) ^2 \right \rvert \text{,}$$ which is just the absolute value of the velocity graph in your screenshot.
This represents the fact that speed is the absolute value of velocity.
We understand "slowing down" to mean that the slope of the speed is negative, and "speeding up" to meantmean that the slope of the speed is positive. What is the slope of point $(6, 0)$ on the graph (which corresponds to your circled dot)?
This point is a cusp. The notion of "slope" only exists for differentiable points, and as Wikipedia says,
a function with a bend, cusp, or vertical tangent may be continuous, but fails to be differentiable at the location of the anomaly.
Thus the slope of speed does not exist at this point, and so the object is neither speeding up nor slowing down in this instant.