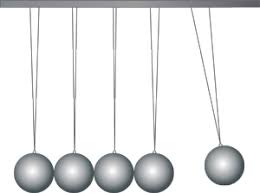
The stopping of the ball which hits another ball depends upon the elasticity of the balls or even one of the two balls. If the balls are very hard like if made of wood or metal, when one ball hits another there is no "waiting time" for the transfer of kinetic energy from one to another. It is near instantaneous and hence one ball transfers nearly all of it's kinetic energy to the ball it hits. Hence the ball "nearly" stops.
Eg. Newtons Cradle
This is not the case in case of elastic balls.
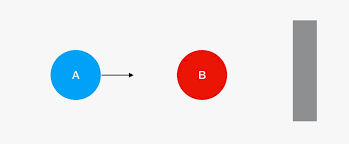
Consider two equal balls of rubber,
When the ball A hits B, the shape of A changes due the inertia of the ball B and also due to it's own elasticity. When the ball A relaxes it transfers it's kinetic energy to the ball B minus the internal friction in the ball A due to shape change and other external frictional losses. Now the ball B deforms a little due to ball A pushing on it(further waste of energy) and moves forward by pushing upon ball A when it relaxes + the energy imparted by the collision itself.
Now the fate of ball A is either it can move forward using the little energy it has left after the collision or roll backwards depending upon the force with which it has hit the other ball.
And it seems even more impossible for Ball 1 to stop completely and Ball 2 to go off at the original velocity of Ball 1.
What am I missing?
If the balls A and B in the above picture were rigid,
The ball B doesn't go off in the original velocity"original velocity", it can be near the original velocity but never the velocity of the ball A itself.
And the ball A stops due to the nearly total transfer of kinetic energy.
Hope this helps.