Your version of $\psi$ is (I'm sure you know) derived from the Time Independent Schrodinger equation, $\hat{H}\psi=E\psi$. To find time-dependent solutions, we solve $$i\frac{\partial}{\partial t}\psi=\hat{H}\psi.$$ You were trying to solve for stationary states, and the entire point of those is that $|\psi(x)|^2$ does not change over time. Still, for these stationary solutions solutions,$$\frac{\mathrm{d}}{\mathrm{d}t}\left<x\right>=\left<p\right>=0; \frac{\mathrm{d}}{\mathrm{d}t}\left<p\right>=-\left<\frac{\mathrm{d}}{\mathrm{d}x}V (x)\right>=0,$$ which is in accordance with the Ehrenfest theorem (albeit uninformatively).
Be careful about the time dependence of your reported $\psi$: you're actually dealing with $\Psi(x, t)=\psi(x)e^{-itE/\hbar}$ for those stationary states. Of course, this doesn't relate to the Ehrenfest theorem, but it's something worth mentioning: the complex and real parts are oscillating, as shown by the pink and blue lines in this diagram (from the wikipedia page on QHO):
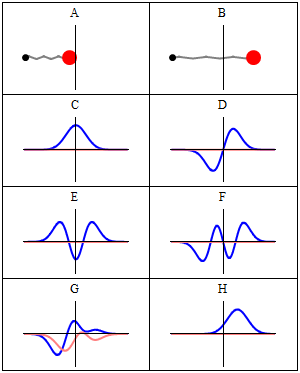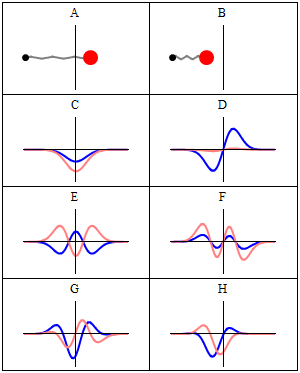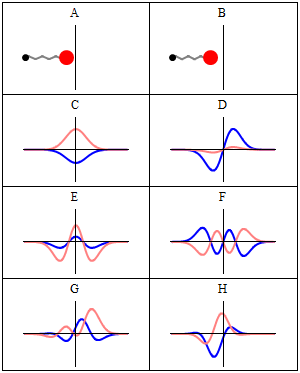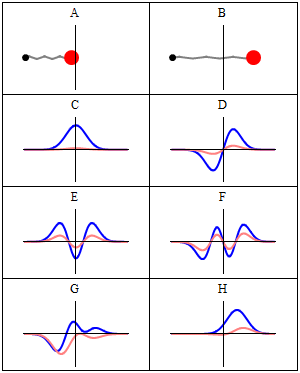
Do not make the mistake of suggesting that theall derivatives with respect to time are necessarily automatically equal to zero because the wavefunction is time-independent. Contrary to what the shorthand notation suggests, we do have a (separable) time-dependent bit.
Referring to the same diagram, observe parts G and H: these represent coherent states, which can be understood using Ehrenfest's theorem because $|\psi|^2$ looks like a Gaussian which follows a classical $\sin$ or $\cos$ function.
See
Kanasugi, H., and H. Okada. “Systematic Treatment of General Time-Dependent Harmonic Oscillator in Classical and Quantum Mechanics.” Progress of Theoretical Physics, vol. 93, no. 5, 1995, pp. 949–960., doi:10.1143/ptp/93.5.949.
