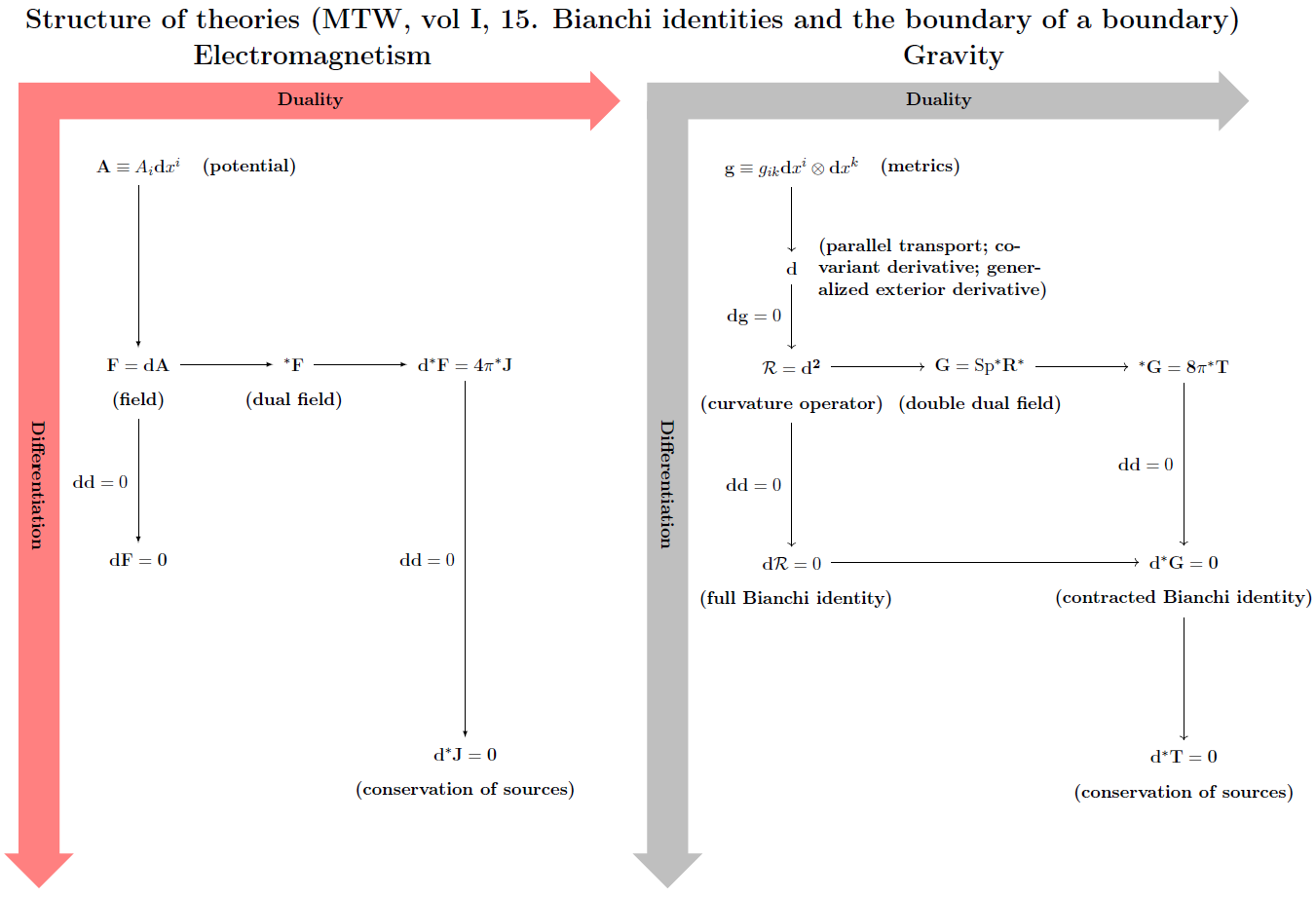
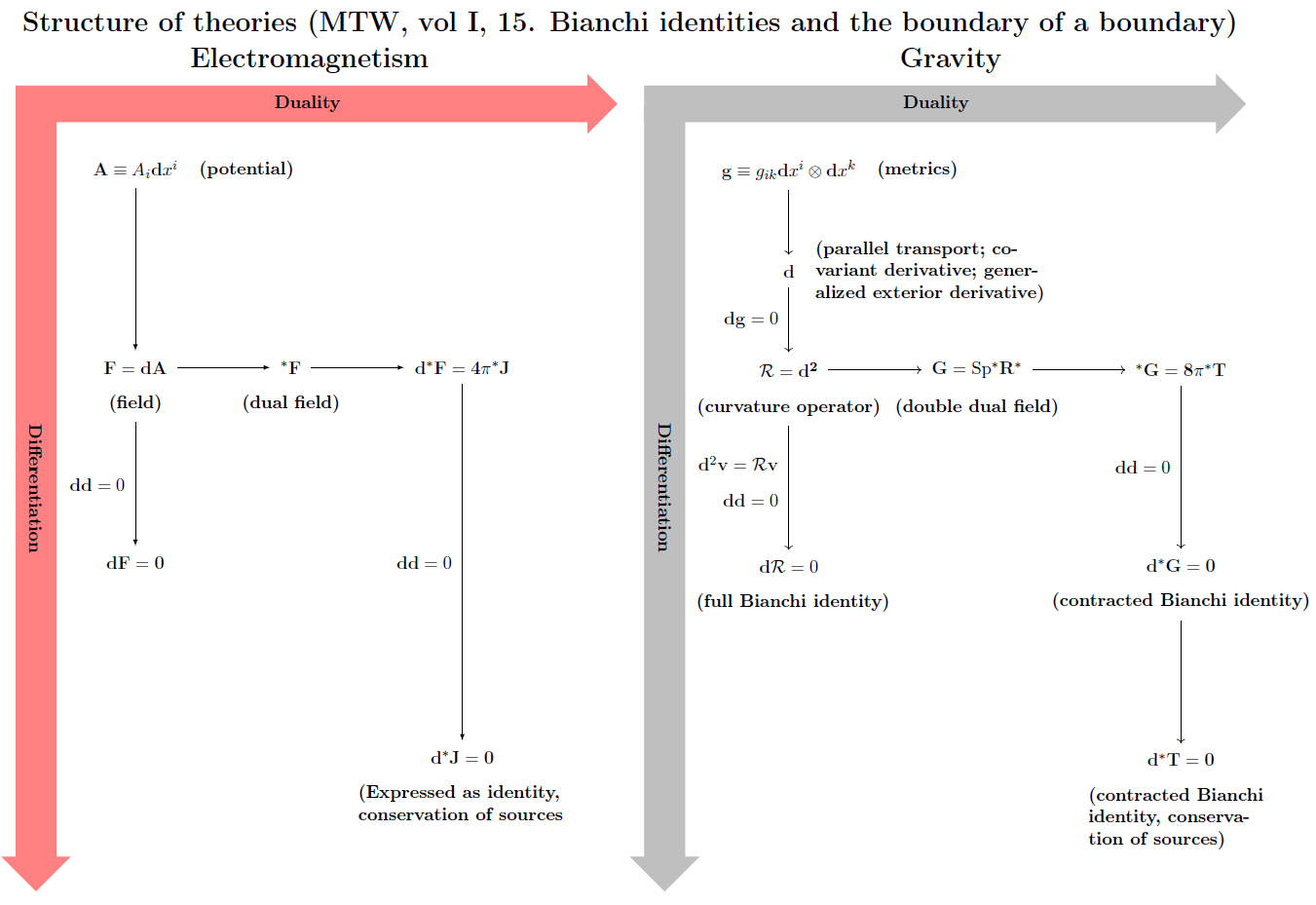
We know, the the vector form of Maxwell equations \begin{align} \vec\nabla\cdot\vec{E} &= 4\pi\rho \label{Diff I}\\ \vec\nabla\times\vec{B} &= \dfrac{4\pi}{c} \vec{j}+\dfrac{1}{c}\dfrac{\partial\vec{E}}{\partial t} \label{Diff IV}\\ \vec\nabla\times\vec{E} &= -\dfrac{1}{c}\dfrac{\partial\vec{B}}{\partial t} \label{Diff III}\\ \vec\nabla\cdot\vec{B} &= 0 \label{Diff II} \end{align}\begin{align} \vec\nabla\cdot\vec{E} &= 4\pi\rho \label{Diff I}\\ \vec\nabla\times\vec{B} &= \dfrac{4\pi}{c} \vec{j}+\dfrac{1}{c}\dfrac{\partial\vec{E}}{\partial t} \label{Diff IV}\\ \vec\nabla\times\vec{E} &= -\dfrac{1}{c}\dfrac{\partial\vec{B}}{\partial t} \label{Diff III}\\ \vec\nabla\cdot\vec{B} &= 0. \label{Diff II} \end{align}
The last two of them allow us to introduce the potentials: \begin{align} \vec{E} &= -\frac1c \frac{\partial \vec{A}}{\partial t} - \vec\nabla\phi\\ \vec{B} &= \vec\nabla\times\vec A \end{align} which tells us about gauge invariance of equations.
All four of Maxwell's equations can be written compactly as
\begin{align} \partial_{\mu}F^{\mu\nu} &= \frac{4\pi}{c}j^{\nu} \tag{1}\\ \partial_{[\mu}F_{\alpha\beta]} &= 0\;. \tag{2} \end{align}
And according to the last one equation, the first one we can rewrite (use preferred gauge) in form: \begin{equation} \Box A^{\mu} = -\frac{4\pi}{c} j^{\mu} \end{equation}\begin{equation} \Box A^{\mu} = -\frac{4\pi}{c} j^{\mu}. \end{equation}
Now we consider the Einstein GR equations: \begin{equation} R_{\mu\nu} = 8\pi G (T_{\mu\nu} - \frac12g_{\mu\nu}T). \end{equation}
Or in "$\Gamma-$field" form (indexes are omitted): \begin{equation} \partial \Gamma - \partial \Gamma + \Gamma\Gamma - \Gamma\Gamma = 8\pi G (T_{\mu\nu} - \frac12g_{\mu\nu}T). \end{equation}
We know, in weak field limit the equations get simply form externally similar to Maxwell ones (so called gravitomagnetism) in 3-vector form, or in covariant form: \begin{equation} \Box h_{\mu\nu} = -16\pi G (T_{\mu\nu} - \frac12\eta_{\mu\nu}T) \end{equation}\begin{equation} \Box h_{\mu\nu} = -16\pi G (T_{\mu\nu} - \frac12\eta_{\mu\nu}T). \end{equation}
Thus, the question: Why for covariant form of Maxwell equations we need two different entities of equations, but for the GR the only one? Or another words, can we write the Einstein GR equations for weak field limit similar to Maxwell equations in field form, not via potentials?