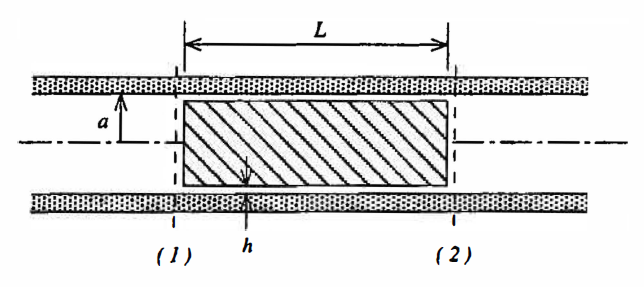
I stumbled upon this exercise in James Fay "Fluid Mechanics" book, which I'm using to learn fluid dynamics by my own, and I am struggling a bit with it, any help will be appreciated:
The figure shows a pig of length $L$ inside a pipe of radius $a$ and having a radial clearance $h<<a$ between its surface and the inner surface of the pipe. When the pressure $P_1$ at $1$ exceeds the pressure $P_2$ at $2$, the pig will move to the right at a constant velocity $V$. Assuming that the flow between the pig and the pipe wall can be con sidered to be a steady plane Couette plus Poiseuille flow in a reference frame attached to the pig.
(a) Derive an expression for the pig velocity V in terms of the parameters $P_1$ , $P_2$, $L$, $h$, $a$ and the fluid viscosity $\nu$. (b) If $Q$ is the volume flow rate of fluid leaking through the clearance gap, relative to the pig, derive an expression for the ratio $Q/\pi a^2V$, which is the ratio of leakage rate to the flow rate of fluid through the pipe.
I have tried solving $a)$ following the tip of using a Couette plus Poiseuille type flow. If I attach a reference frame to the pig (its x axis located at the surface of the pig and parallel the cylinder axis, and the y axis perpendicular to it), we see that the no-slip condition means that $u(y=0)=0$. Moreover, we see that at $y=h$, $u(h)=V$, where $V$ is the magnitude of the velocity of the pig in the laboratory frame. It can be proved that the velocity of the fluid within the pig-wall gap is:
$$u(y)=V\frac{y}{h}+\frac{1}{2\mu}(-\frac{dp}{dx})y(h-y)$$
Now, if I understand correctly, the problem asks me to obtain $V$ as a function of the aforementioned parameters. However, I am stuck in this step as after isolating $V$ I have no idea on how to get rid of the $u$ dependence of it. I can eliminate the $\frac{dP}{dx}$ term by noticing that this is just $\frac{P_2-P_1}{L}$ but that's it.
Integrating in y to get the ratio $\frac{Q}{W}$ yields:
$$\frac{Q}{W}=\frac{Vh}{2}+\frac{h^3}{12\mu}(\frac{P_2-P_1}{L})$$
So $V=\frac{2Q}{hW}-\frac{h^2}{6\mu}(\frac{P_2-P_1}{L})=\frac{Q}{h\pi a}-\frac{h^2}{6\mu}(\frac{P_2-P_1}{L})$