One has to think quantum mechanically. In quantum mechanics there are probability distributions given by the square of the complex wavefunction ofevaluated with the boundary conditions of the specific problem at hand.
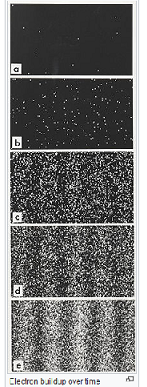
The double slit is the scattering problem " electron scattering on two slits of given width and distance between them" . The single electron is a spot on the screen, the footprint of a particle similar to a classical particle, an (x,y,z).
The spot looks random, it is only the probability distribution accumulated over many electrons that displays a wave nature.
Moreover if we install a detector at the slits, surprisingly the interference pattern vanishes (which explains wave-particle complementarity) but What tells the electron that it is being watched?
It is the change in the boundary conditions, which detecting which slit the electron went through necessarily induces, that changes the probability distribution. It is a different experiment.