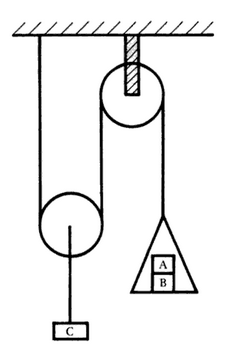
Can anyone please help me with the following problem?
Each of the weights A and B has a mass M and C has a mass of kM.
A) If the system is released form rest find the acceleration of the moveable pulley and the scale pan and show that the scale pan will ascend if k > 4.
B) When the system is moving freely find the tension in the string.
It is part B that is confusing me. In fact I don't need to solve part A to solve part B, but whether I use my answer from part A or not I get the same answer for part B which is different from the text book answer.
Here's my solution for part A:
I set the acceleration of the pan to a in a downwards direction.
Then the acceleration of C in the upward direction will be a/2, as the string to the left of C is not accelerating and the string to the right of C is accelerating at a.
For C:
$2T-kmg=ma/2$
For pan:
$2mg-T=2mga$
Now I do the algebra to eliminate T and arrive at:
$a=\frac{2g(4-k)}{8g+1}$
Great, if k > 4 then a is negative and so pan ascends - as was to be shown.
For part B I could substitute my value of a into one of the original two equations, or simply eliminate a from those original two equations.
Doing the latter:
$a=\frac{2mg-T}{2mg}$
Therefore
$8gt-4kmg^2=2mg-T$
This leads to:
$T=\frac{2mg(1+2kg)}{8g+1}$
But the book answer is:
$T=\frac{6kmg}{k+8}$
Which is not even close to mine.
Thanks for any help, Mitch.