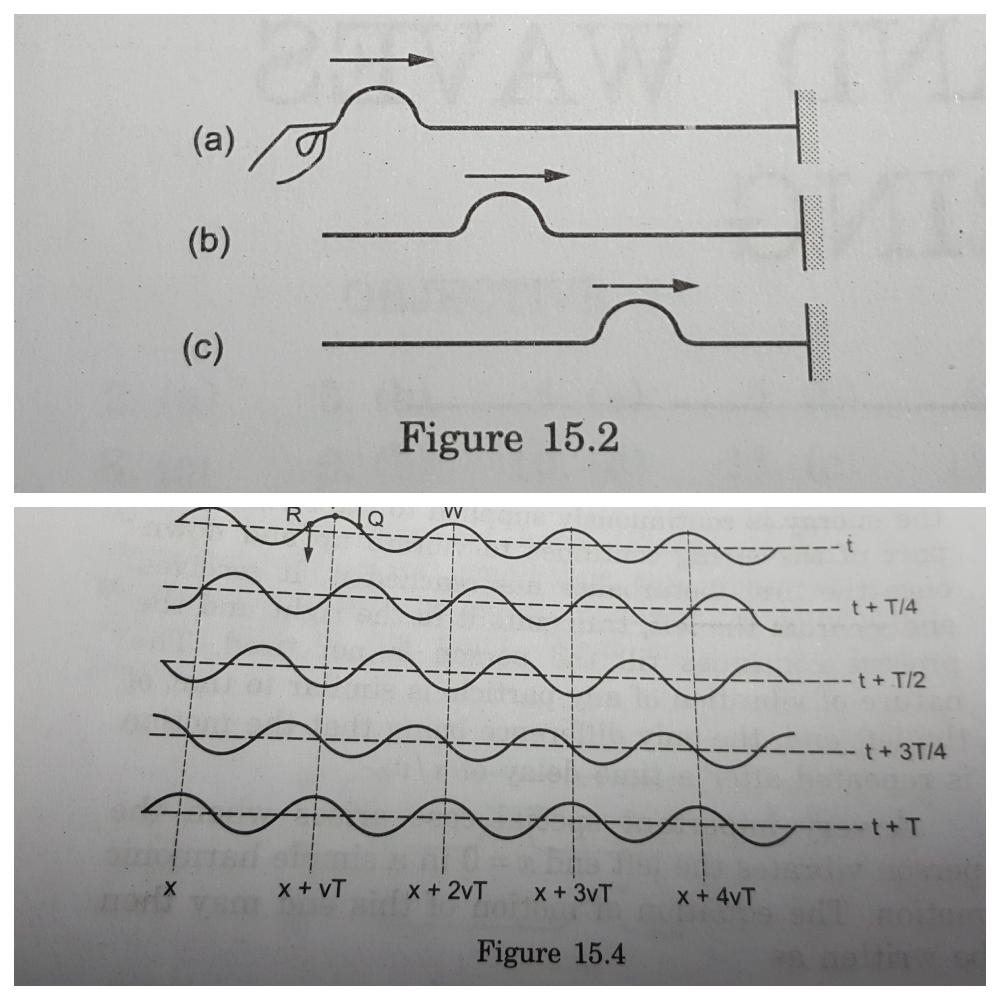
I'd like to describe a scenario in which a wave pulse propagates on a string.
Consider a long string with one end fixed to a wall and the other held by a person. Suppose the person snaps his hand a little up and down producing a bump in the string near his hand. The operation takes a very small time, say one tenth of a second after which the person still stands holding the string in his hand.
Now, I want to proceed with defining wave pulse on string. When a disturbance is localised only to a small part of the space at a time, we say that a wave pulse is passing through that part of the space. This happens when the source producing the disturbance (hand in this case) is active only for a short time.
If the source is active for some extended time repeating its motion several times, we get a wave train or a wave packet. For example, if the person in figure 15(15.2) decides to vibrate his hand up and down 10 times and then stop, a wave train consisting of 10 loops will proceed on the string. The last line for me is a problem when analysing a sinusoidal wave. If the person holding the string in figure 15(15.2) keeps waving his hand up and down continuously, he keeps doing work on the string and the energy is continuously supplied to the string. A very important special case arises when the person vibrates the left end in a simple harmonic motion. The wave produced by such a vibrating source is called a sinusoidal wave.
I am unable to understand how this wave train propagates on a string. Obviously, a sine wave on a string will produce a wave train as the source producing the disturbance is not active only for a short time. How will this wave train propagate?. I am unable to figure out how this wave train moves on a string.
I thought that a certain number of "bumps" will move on the string in the same fashion even for a wave train. How does it move as shown in figure 15(15.4). Also, how does the left end and right end keep changing as time progresses?. In figure 15(15.2), it was just one small bump (the bump never changed) and the same bump moved on the string with speed $v$. How does the configuration of the sine wave keep changing with time and how does it propagate with speed $v$ on the string. For clarity, I would pose my question as:
How does a sine wave / sinusoidal wave propagate on a string. Since its configuration changes with time (as shown in the figure), then how does it move with speed $v$ on the string by changing its configuration at the same time. For a wave pulse, it was just one configuration and it kept moving with constant speed. Obviously the particles of sine wave keep executing simple harmonic motion vertically, but then how does the wave propagate and move forward with speed $v$?