I'm conducting a X-ray diffraction experiment for lab, but am new to solid-state physics and crystallography. I'mI have to find the Debye-Waller factor (DWF) of Al at room temperature using X-ray powder diffraction. The value given in the International Tables for X-Ray Crystallography Vol. 3 is approximately $0.78 \mathring{A}^{2}$.
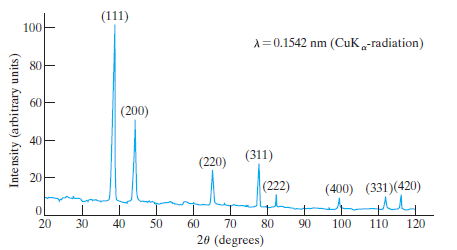
I obtain the diffraction peaks using a diffractometer with $\text{Cu}K_{\alpha}$ radiation and a Ni filter at the receiving slit.
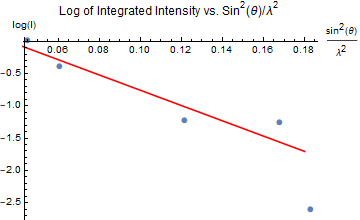
I find the integrated intensity $I$ relative to that of the first diffraction peak by finding the peaks' areas above the background. Since $$I=K\exp(-2B\sin^2\theta/\lambda^2)$$ then $$\log I=\log K-2B\frac{\sin^2\theta}{\lambda^2}$$ where $\theta$ is the diffraction angle, $\lambda$ the wavelength in angstrom of the radiation incident on the aluminum powder sample, and $B$ is the DWF. $K$ is a constant proportional to factors like the structure factor and Lorentz polarization factors. I then fit the plot of $\log I$ vs. $\frac{\sin^2\theta}{\lambda^2}$ with a linear function, and divide the slope of the fit by $2$ to get the DWF.
The diffraction peaks I'm seeing are extremely similar to the plot of the diffraction peaks below:
However, when I actually plot $\log I$ vs. $\sin^2\theta/\lambda^2$, I get:
and a DWF of $5.92 \mathring{A}^{2}$. Due to this difference in magnitude, I feel like I'm doing something incorrectly when measuring the integrated intensity. Could someone offer a suggestion?
Thank you in advance.