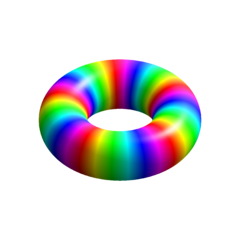A stationary state is not the same thing as a standing wave.
The wave functions that come closest to the deBroglie picture are "circular orbitals", states with large principal quantum number $n$ and maximum value of angular momentum $\ell = n-1$. When then $m_\ell = \pm \ell$, the phase of the wave function varies only as a function of the angle in the plane of the orbital. Here an image for $n=6$ where phase is coded as color:
This is the time-independent part of the wave function. There are five deBroglie wavelengths around the circle, $\ell = 5.$ To get the time-dependent wave function, one multiplies with the phase factor $e^{iEt/\hbar}$.$e^{iEt/\hbar}.$ This adds a phase that grows with time, so that the colors rotate.
A standing wave would be a sum (or the difference) of the clockwise and the anti-clockwise pattern, $m_\ell = \ell$ and $m_\ell = - \ell$$m_\ell = - \ell.$