This is from John Taylor- "An Introduction to Error Analysis"
The passage asks us to calculate the uncertainty of refractive index n using Snell's Law
$$\sin i=n \sin r$$
The fractional uncertainty in $n$ is the quadratic sum of those in $\sin i$ and $\sin r$:
$$\frac{\delta n}{n}=\sqrt{\left(\frac{\delta \sin i}{\sin i} \right)^2+\left(\frac{\delta \sin i}{\sin r}\right)^2}$$$$\frac{\delta n}{n}=\sqrt{\left(\frac{\delta \sin i}{\sin i} \right)^2+\left(\frac{\delta \sin r}{\sin r}\right)^2}$$
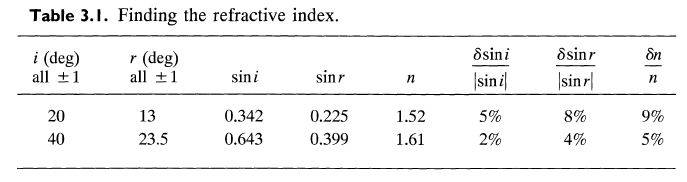
The calculation is then carried out as shown in the table below
And then in the last paragraph, the author mentioned that"If you repeat an experiment like this one several times, the error calculations can become tedious if you do them for each repetition. You should recognize, however, that you almost never need to do the error calculations for all the repetitions; if you find the uncertainties in $n$ corresponding to the smallest and largest values of $i$ (and possibly a few intermediate values), then these uncertainties suffice for most purposes."
I don't understand why don't we need to do the error calculations for all the repetitions, since the $\frac{\delta n}{n}$ values look quite different for each repetition in the table? And what does he mean by "if you find the uncertainties in $n$ corresponding to the smallest and largest values of $i$"?
Really appreciate the help!