My question pertains to the following excerpt from my lecture notes:
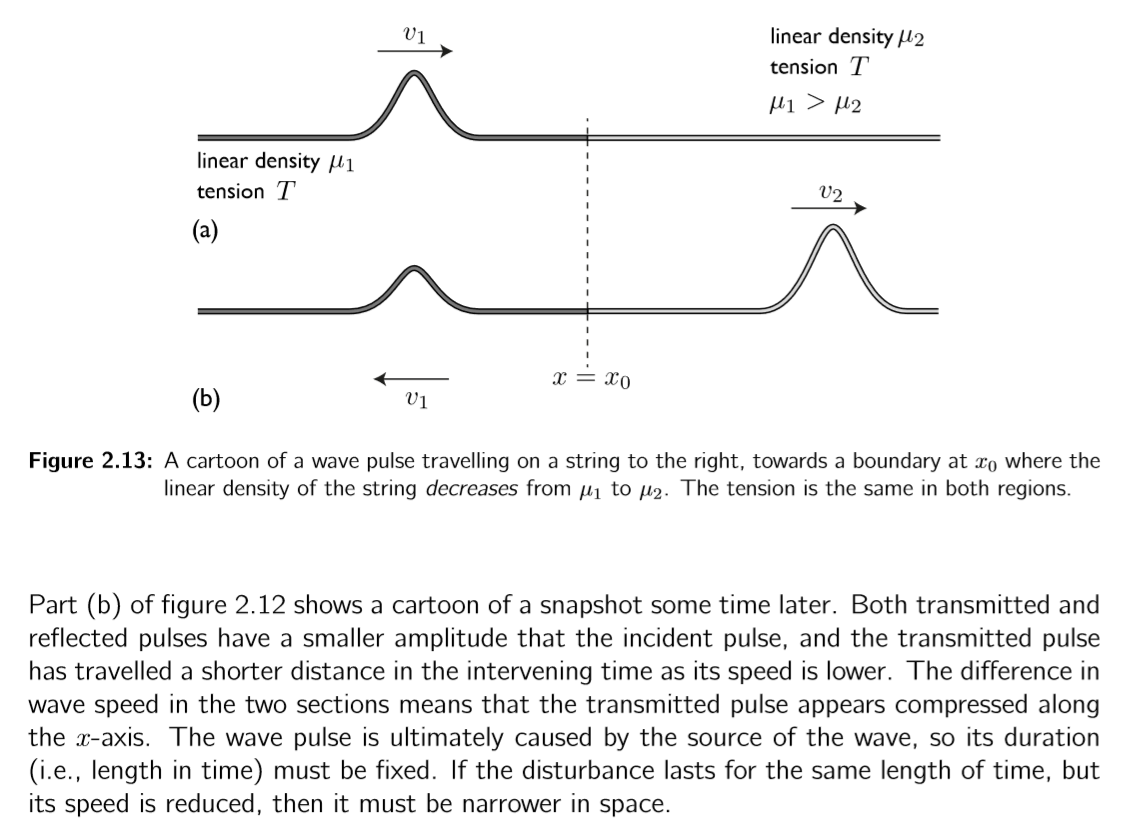
Part (b) of figure 2.12 shows a cartoon of a snapshot some time later. Both transmitted and reflected pulses have a smaller amplitude that the incident pulse, and the transmitted pulse has travelled a shorter distance in the intervening time as its speed is lower. The difference in wave speed in the two sections means that the transmitted pulse appears compressed along the x-axis. The wave pulse is ultimately caused by the source of the wave, so its duration (i.e., length in time) must be fixed. If the disturbance lasts for the same length of time, but its speed is reduced, then it must be narrower in space.
I have trouble interpreting why the transmitted wave is narrower in space.
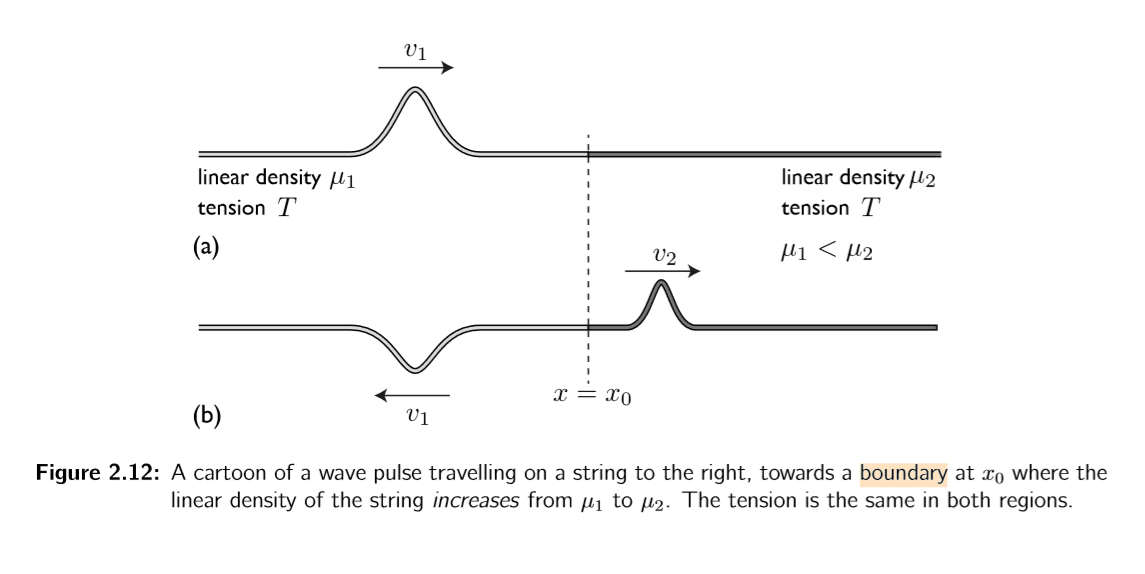
Firstly, I don't see how the transmitted pulse has traveled a shorter distance in the intervening time as its speed is lower - here, the linear density in which the transmitted pulse moves in is less than that of the reflected pulse, so how can its speed be lower?
Secondly, I don't understand "the wave pulse is ultimately caused by the source of the wave, so its duration must be fixed". Yes, it's caused by the source of the wave, but where does this then imply the duration is fixed?
Thus, it is stated "If the disturbance lasts for the same length of time, but its speed is reduced, then it must be narrower in space." However, I haven't accepted the first two points because I don't see how they make sensethese two points imply the third.
Can someone help in illuminating this for me?