I'm really rusty on my special relativity, and could use some help on this problem. (Doing for fun, not homework.) Thanks.
Here's a space-time diagram with c=1 units:
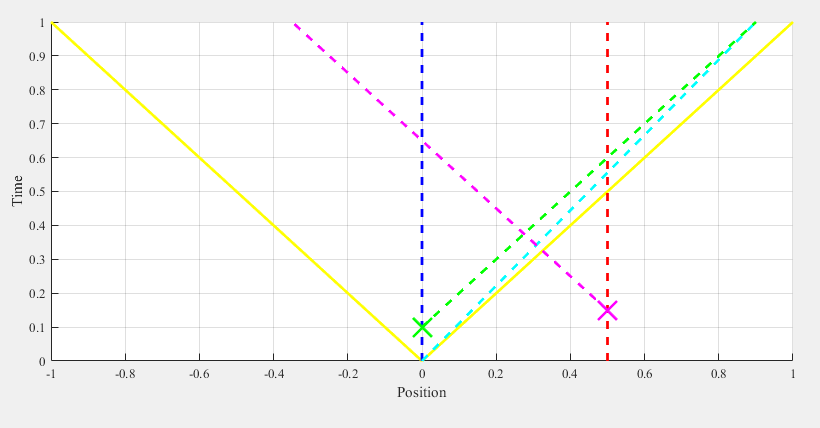
Yellow Lines: Light Cones
Blue & Red Vertical Lines: Planets (at x = 0 & 0.5)
Cyan Line: Space Ship moving at 0.9
Green & Magenta X's: Events that happen on each planet (t = 0.10 & 0.15)
Green & Magenta Lines: The light traveling from the event
I'm trying to transform this diagram into one that is in the reference frame of the space ship, but I'm struggling with my Lorentz boosts. I know the length should contract between the planets:
$$ L' = L/\gamma $$
I know I should be using the Lorentz equations
$$ t' = \gamma (t - vx) \\ x' = \gamma (x - vt) $$
but when I try these, I get weird answers. For example, when I try to calculate the time of the magenta event in the reference frame of the spaceship:
$$ t' = \frac{1}{1 - 0.9^2}(0.15 - 0.9 * 0.5) = -0.69 $$
which is really far in the past, as far as these events are concerned.
Can someone help me with how to transform this? Specifically:
Am I doing the contraction right between the planets?
Should the planets be seen moving away at a speed of 0.9, or does that change?
How do I calculate the timing of the events in the new coordinate frame where the spaceship is at rest?
Thank you so much for your help.
