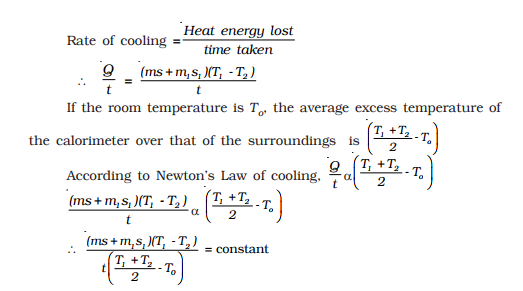
I am reading about Newton's Law of Cooling and the following is an extract from my textbook on the experimental verification of this law.
"Consider a spherical calorimeter of mass m whose outer surface is blackened. It is filled with hot water of mass m1. The calorimeter with a thermometer is suspended from a stand. The calorimeter and the hot water radiate heat energy to the surroundings. Using a stop clock, the temperature is noted for every 30 seconds interval of time till the temperature falls by about 20o C. The readings are entered in a tabular column.
If the temperature of the calorimeter and the water falls from T1 to T2 in t seconds, the quantity of heat energy lost by radiation Q = (ms + m1s1) (T1 – T2), where s is the specific heat capacity of the material of the calorimeter and s1 is the specific heat capacity of water."
I have the following doubts:
How can the temperature of both the water and the calorimeter fall from temperature T1 to T2 ? If the initial temperature of the hot water is T1, then is it assumed that the temperature of the calorimeter is also T1 ? Even so, won't their rates of cooling be different ?
What exactly is the " mean excess temperature" ? This is the definition I found online:
"Excess Temperature is defined as the temperature difference between heat source and saturation temperature of the fluid."
But my textbook says nothing about this 'saturation temperature'.
I apologize if this is too long. But I couldn't find much information online....