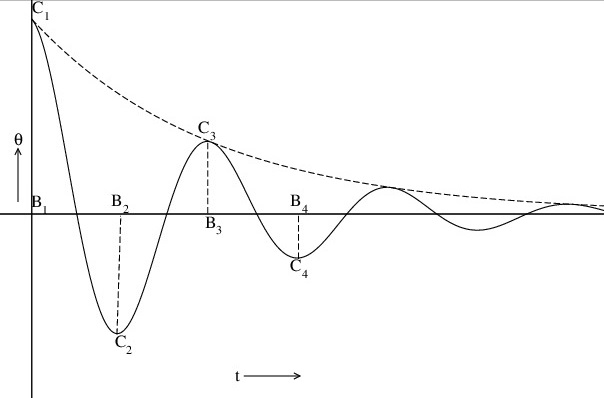
I have attached an image of a sinusoidal damped oscillation that shows the two amplitudes of which ratio x = B 1 C 1 / B 2 C 2$x = B_1 C_1 / B_2 C_2$ {( x 1 / x 2which is )in$x_1 / x_2$ in the question}) is measured
hereHere,
 $$
x = e^\lambda = \frac{B_1C_1}{B_2C_2} = \frac{B_2C_2}{B_3C_3}
$$
$$
x = e^\lambda = \frac{B_1C_1}{B_2C_2} = \frac{B_2C_2}{B_3C_3}
$$
xand $x$ is what is named decrement. In
In order to usually find the viscosity of the medium in which a pendulum oscillates and it's amplitude studied (or any other purpose), we define a term called logarithmic decrement,λ $λ$ that is numerically equal to log(x).
Do give feedback on the answer (my first) and ask clarification anywhere if required$\log(x)$.