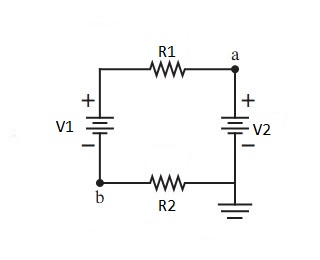
Consider the following circuit consisting of 2 batteries and 2 resistors.
How would I find the value of the potential at points $a$ and $b$?
In my initial attempt I assumed that the value of the current was consistent throughout the loop (which is where I think I went wrong) and tried to use Kirchoff's Loop Rule, giving, but seeing as there are no nodes I'm not sure how to go about solving it after.
Additional Info Edit: Using Kirchoff's, I thus far found:
V1 - R1I - V2 - R2I = 0
Solving for intensitycurrent:
I = (V1 - V2) / (R1 + R2)
And so the potential difference across is R1 is V = R1I = R1(V1 - V2) / (R1 + R2).
So the potential at a would be V1 - R1*(V1 - V2) / (R1 + R2).