Note: Additional material added at the bottom.
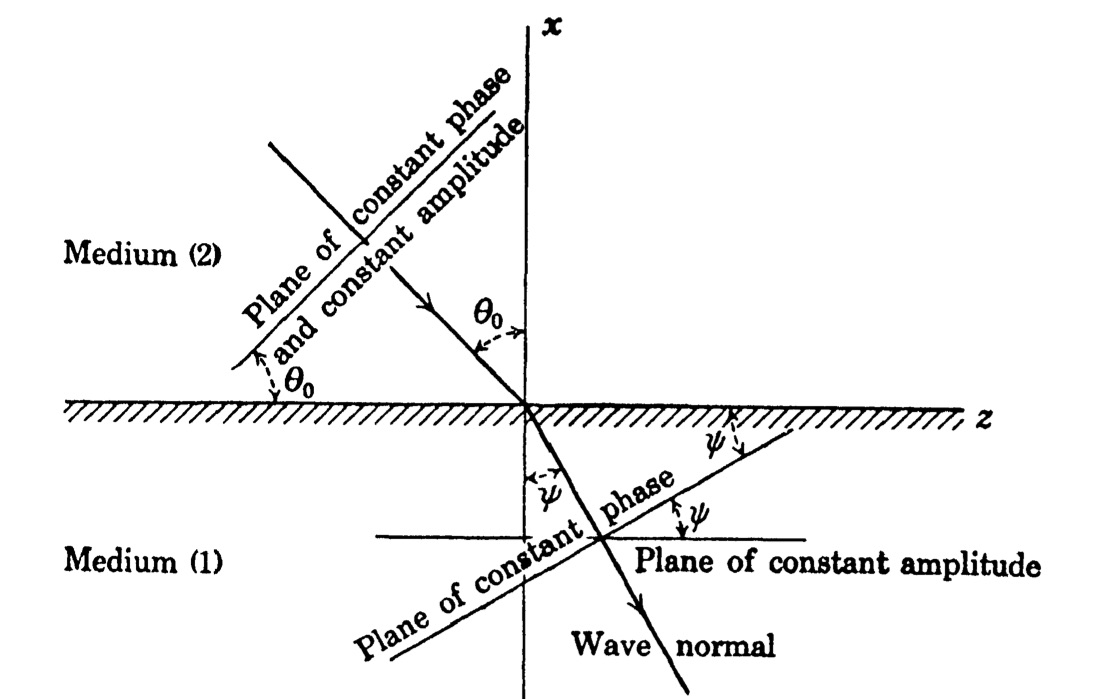
As far as I can tell, the electric and magnetic fields are always perpendicular (at least in linear media, which appears to be implied by the question). However, in lossy media, it is possible that direction of propagation - as defined by the direction perpendicular to a plane of constant phase - not be perpendicular to $\vec H$. This is illustrated in the figure below, taken from Stratton, Julius Adams, Electromagnetic theory, McGraw-Hill (1941).
In the figure, medium (2) is a lossless medium whereas medium (1) is lossy. For an incident electric field along $\hat y$, the transmitted field in medium (1) is of the form $$ \vec E_t(x,z)= \hat y E_{t0} e^{-p z} e^{-i (x\beta_2\sin\theta_2+qz)} $$ In medium (1), the planes of constant amplitude ($pz=$ constant) do not align with the planes of constant phase, which are defined by $$ \vec k_\psi\cdot \vec r=\hbox{constant}=qz+\beta_2 x \sin\theta_2\, . $$ The expression for the transmitted magnetic field is $$ \vec H_t(x,z)=\frac{E_{t0}}{\omega\mu_0}\left(\hat x i(p+iq)+\hat z \beta_2\sin\theta_2\right)e^{-p z} e^{-i (x\beta_2\sin\theta_2+qz)} $$ and one can verify that $\vec k_\psi\cdot \vec H_t\ne 0$.
Beyond Stratton cited above, another good source for this is: U.S. Inan, A.S. Inan and R. K. Said, Engineering Electromagnetics and waves, Pearson (2015)
Additional elements to my original answer
After some research it seems that $\vec E$ and $\vec B$ are always perpendicular, at least in lossless media, even when the material is electrically anisotropic. This can be guessed from $$ \vec\nabla\times \vec E= -\frac{\partial}{\partial t}\vec B\, . $$
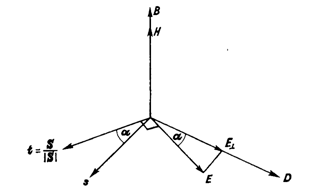
In an electrically-anisotropic but magnetically isotropic medium , $\vec H$ and $\vec B$ are parallel, but $\vec D$ need not be parallel to $\vec E$. Nevertheless, the pair $\vec D$ and $\vec E$ are perpendicular to $\vec B$ or $\vec H$. This is illustrated in the figure below, extracted from Chapter XIV of
- Born, Max, and Emil Wolf. Principles of optics: electromagnetic theory of propagation, interference and diffraction of light. Elsevier, 2013.
In the figure, $\hat s$ is the direction of propagation in the sense that $\vec E\sim e^{i\omega \left(\frac{n}{c}(\vec r\cdot \vec s-t)\right)}$ and similarly for $\vec D, \vec H$ and $\vec B$. The direction $\hat t=\vec S/\vert \vec S\vert$ (somewhat unfortunate choice of symbol) is the direction of the Poynting vector, i.e. the direction of propagation of energy (which is not aligned with the direction of propagation).
There is a footnote in this chapter that states, part of which reads
There are also magnetic crystals, but as the effect of magnetization on optical phenomena (rapid oscillations) is small, the magnetic anisotropy may be neglected.
When the material is lossy, we go back to the original part of my answer.